Advertisements
Advertisements
Question
If f : R → R is defined by f(x) = x2, write f−1 (25)
Solution
Let f−1 (25) =x ... (1)
⇒ f (x) = 25
⇒ x2 = 25
⇒ x2 − 25 = 0
⇒ (x−5) (x+5) = 0
⇒ x = ±5
⇒ f−1 (25) = {−5, 5} [from (1)]
APPEARS IN
RELATED QUESTIONS
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x3
Let A = R − {3} and B = R − {1}. Consider the function f: A → B defined by `f(x) = ((x- 2)/(x -3))`. Is f one-one and onto? Justify your answer.
Give examples of two functions f: N → Z and g: Z → Z such that g o f is injective but gis not injective.
(Hint: Consider f(x) = x and g(x) =|x|)
Let A = {−1, 0, 1} and f = {(x, x2) : x ∈ A}. Show that f : A → A is neither one-one nor onto.
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 5x3 + 4
Let A = [-1, 1]. Then, discuss whether the following functions from A to itself is one-one, onto or bijective : h(x) = x2
Set of ordered pair of a function? If so, examine whether the mapping is injective or surjective :{(x, y) : x is a person, y is the mother of x}
If f : R → R be the function defined by f(x) = 4x3 + 7, show that f is a bijection.
If A = {1, 2, 3}, show that a one-one function f : A → A must be onto.
If A = {1, 2, 3}, show that a onto function f : A → A must be one-one.
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 2x + x2 and g(x) = x3
Let f = {(3, 1), (9, 3), (12, 4)} and g = {(1, 3), (3, 3) (4, 9) (5, 9)}. Show that gof and fog are both defined. Also, find fog and gof.
Find fog (2) and gof (1) when : f : R → R ; f(x) = x2 + 8 and g : R → R; g(x) = 3x3 + 1.
Consider f : N → N, g : N → N and h : N → R defined as f(x) = 2x, g(y) = 3y + 4 and h(z) = sin z for all x, y, z ∈ N. Show that ho (gof) = (hog) of.
Find fog and gof if : f (x) = |x|, g (x) = sin x .
If f(x) = sin x and g(x) = 2x be two real functions, then describe gof and fog. Are these equal functions?
if f (x) = `sqrt (x +3) and g (x) = x ^2 + 1` be two real functions, then find fog and gof.
If f, g : R → R be two functions defined as f(x) = |x| + x and g(x) = |x|- x, ∀x∈R" .Then find fog and gof. Hence find fog(–3), fog(5) and gof (–2).
A function f : R → R is defined as f(x) = x3 + 4. Is it a bijection or not? In case it is a bijection, find f−1 (3).
If f : R → (−1, 1) defined by `f (x) = (10^x- 10^-x)/(10^x + 10 ^-x)` is invertible, find f−1.
If f : C → C is defined by f(x) = x4, write f−1 (1).
If f(x) = x + 7 and g(x) = x − 7, x ∈ R, write fog (7).
If f(x) = 4 −( x - 7)3 then write f-1 (x).
The range of the function
\[f\left( x \right) =^{7 - x} P_{x - 3}\]
Which of the following functions from
to itself are bijections?
Let \[f\left( x \right) = x^2 and g\left( x \right) = 2^x\] Then, the solution set of the equation
Consider the set A containing n elements. Then, the total number of injective functions from A onto itself is ______
Let f: `[2, oo)` → R be the function defined by f(x) = x2 – 4x + 5, then the range of f is ______.
Let f: R → R be given by f(x) = tan x. Then f–1(1) is ______.
If f(x) = (4 – (x – 7)3}, then f–1(x) = ______.
The number of bijective functions from set A to itself when A contains 106 elements is ____________.
Which of the following functions from Z into Z is bijective?
The function f : R → R given by f(x) = x3 – 1 is ____________.
Let f : R → R, g : R → R be two functions such that f(x) = 2x – 3, g(x) = x3 + 5. The function (fog)-1 (x) is equal to ____________.
The solution set of the inequation log1/3(x2 + x + 1) + 1 > 0 is ______.
`x^(log_5x) > 5` implies ______.
Consider a set containing function A= {cos–1cosx, sin(sin–1x), sinx((sinx)2 – 1), etan{x}, `e^(|cosx| + |sinx|)`, sin(tan(cosx)), sin(tanx)}. B, C, D, are subsets of A, such that B contains periodic functions, C contains even functions, D contains odd functions then the value of n(B ∩ C) + n(B ∩ D) is ______ where {.} denotes the fractional part of functions)
If f : R `rightarrow` R is defined by `f(x) = (2x - 7)/4`, show that f(x) is one-one and onto.
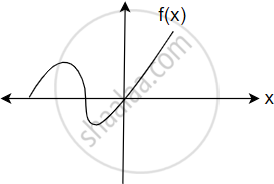
The given function f : R → R is not ‘onto’ function. Give reason.
