Advertisements
Advertisements
Question
If f(x) = x + 7 and g(x) = x − 7, x ∈ R, write fog (7).
Solution
\[\left( fog \right)\left( 7 \right) = f \left( g\left( 7 \right) \right)\]
\[ = f\left( 7 - 7 \right)\]
\[ = f \left( 0 \right)\]
\[ = 0 + 7\]
\[ = 7\]
APPEARS IN
RELATED QUESTIONS
Check the injectivity and surjectivity of the following function:
f: N → N given by f(x) = x3
Show that the modulus function f: R → R given by f(x) = |x| is neither one-one nor onto, where |x| is x, if x is positive or 0 and |x| is − x if x is negative.
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f: R → R defined by f(x) = 1 + x2
Let f: R → R be defined as f(x) = 10x + 7. Find the function g: R → R such that g o f = f o g = 1R.
Show that function f: R `rightarrow` {x ∈ R : −1 < x < 1} defined by f(x) = `x/(1 + |x|)`, x ∈ R is one-one and onto function.
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Classify the following function as injection, surjection or bijection : f : Z → Z given by f(x) = x3
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = sin2x + cos2x
If A = {1, 2, 3}, show that a onto function f : A → A must be one-one.
Find the number of all onto functions from the set A = {1, 2, 3, ..., n} to itself.
Let R+ be the set of all non-negative real numbers. If f : R+ → R+ and g : R+ → R+ are defined as `f(x)=x^2` and `g(x)=+sqrtx` , find fog and gof. Are they equal functions ?
If f : A → B and g : B → C are onto functions, show that gof is a onto function.
Find f −1 if it exists : f : A → B, where A = {0, −1, −3, 2}; B = {−9, −3, 0, 6} and f(x) = 3 x.
Consider f : {1, 2, 3} → {a, b, c} and g : {a, b, c} → {apple, ball, cat} defined as f (1) = a, f (2) = b, f (3) = c, g (a) = apple, g (b) = ball and g (c) = cat. Show that f, g and gof are invertible. Find f−1, g−1 and gof−1and show that (gof)−1 = f −1o g−1
If f : Q → Q, g : Q → Q are two functions defined by f(x) = 2 x and g(x) = x + 2, show that f and g are bijective maps. Verify that (gof)−1 = f−1 og −1.
Let f : R `{- 4/3} `- 43 →">→ R be a function defined as f(x) = `(4x)/(3x +4)` . Show that f : R - `{-4/3}`→ Rang (f) is one-one and onto. Hence, find f -1.
Let f be a function from C (set of all complex numbers) to itself given by f(x) = x3. Write f−1 (−1).
If f : R → R is defined by f(x) = 3x + 2, find f (f (x)).
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. State whether f is one-one or not.
Let A = R − (2) and B = R − (1). If f: A ⟶ B is a function defined by`"f(x)"=("x"-1)/("x"-2),` how that f is one-one and onto. Hence, find f−1.
Let f: R → R be defined by f(x) = x2 + 1. Then, pre-images of 17 and – 3, respectively, are ______.
Let D be the domain of the real valued function f defined by f(x) = `sqrt(25 - x^2)`. Then, write D
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(a, b): a is a person, b is an ancestor of a}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
g = {(1, 4), (2, 4), (3, 4)}
Using the definition, prove that the function f: A→ B is invertible if and only if f is both one-one and onto
Let f: R – `{3/5}` → R be defined by f(x) = `(3x + 2)/(5x - 3)`. Then ______.
Let f: R → R be given by f(x) = tan x. Then f–1(1) is ______.
Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1,2,3,4,5,6}. Let A be the set of players while B be the set of all possible outcomes.
A = {S, D}, B = {1,2,3,4,5,6}
- Raji wants to know the number of functions from A to B. How many number of functions are possible?
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.
Answer the following questions using the above information.
- Let f: {1,2,3,....} → {1,4,9,....} be defined by f(x) = x2 is ____________.
If `f : R -> R^+ U {0}` be defined by `f(x) = x^2, x ∈ R`. The mapping is
Let n(A) = 4 and n(B) = 6, Then the number of one – one functions from 'A' to 'B' is:
If log102 = 0.3010.log103 = 0.4771 then the number of ciphers after decimal before a significant figure comes in `(5/3)^-100` is ______.
Number of integral values of x satisfying the inequality `(3/4)^(6x + 10 - x^2) < 27/64` is ______.
Let x is a real number such that are functions involved are well defined then the value of `lim_(t→0)[max{(sin^-1 x/3 + cos^-1 x/3)^2, min(x^2 + 4x + 7)}]((sin^-1t)/t)` where [.] is greatest integer function and all other brackets are usual brackets.
Let f: R→R be a polynomial function satisfying f(x + y) = f(x) + f(y) + 3xy(x + y) –1 ∀ x, y ∈ R and f'(0) = 1, then `lim_(x→∞)(f(2x))/(f(x)` is equal to ______.
Let f(x) be a polynomial of degree 3 such that f(k) = `-2/k` for k = 2, 3, 4, 5. Then the value of 52 – 10f(10) is equal to ______.
A function f : [– 4, 4] `rightarrow` [0, 4] is given by f(x) = `sqrt(16 - x^2)`. Show that f is an onto function but not a one-one function. Further, find all possible values of 'a' for which f(a) = `sqrt(7)`.
If f : R `rightarrow` R is defined by `f(x) = (2x - 7)/4`, show that f(x) is one-one and onto.
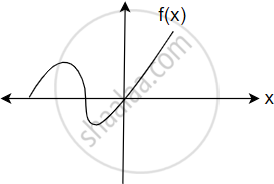
The given function f : R → R is not ‘onto’ function. Give reason.
