Advertisements
Advertisements
Question
Let A = {−1, 0, 1} and f = {(x, x2) : x ∈ A}. Show that f : A → A is neither one-one nor onto.
Solution
A = {−1, 0, 1} and f = {(x, x2) : x ∈ A}
Given, f(x) = x2
Injectivity :
f(1) = 12=1 and
f(-1)=(-1)2=1
⇒ 1 and -1 have the same images.
So, f is not one-one.
Surjectivity :
Co-domain of f = {-1, 0, 1}
f(1) = 12 = 1,
f(-1) = (-1)2 = 1 and
f(0) = 0
⇒ Range of f = {0, 1}
So, both are not same.
Hence, f is not onto
APPEARS IN
RELATED QUESTIONS
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x3
Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
F = {(a, 3), (b, 2), (c, 1)}
Give an example of a function which is neither one-one nor onto ?
Which of the following functions from A to B are one-one and onto?
f1 = {(1, 3), (2, 5), (3, 7)} ; A = {1, 2, 3}, B = {3, 5, 7}
Which of the following functions from A to B are one-one and onto?
f2 = {(2, a), (3, b), (4, c)} ; A = {2, 3, 4}, B = {a, b, c}
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = sin2x + cos2x
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 1 + x2
Show that if f1 and f2 are one-one maps from R to R, then the product f1 × f2 : R → R defined by (f1 × f2) (x) = f1 (x) f2 (x) need not be one - one.
Find gof and fog when f : R → R and g : R → R is defined by f(x) = x and g(x) = |x| .
Let A = {a, b, c}, B = {u v, w} and let f and g be two functions from A to B and from B to A, respectively, defined as :
f = {(a, v), (b, u), (c, w)}, g = {(u, b), (v, a), (w, c)}.
Show that f and g both are bijections and find fog and gof.
Find fog and gof if : f (x) = x+1, g (x) = sin x .
Let f be any real function and let g be a function given by g(x) = 2x. Prove that gof = f + f.
If f, g : R → R be two functions defined as f(x) = |x| + x and g(x) = |x|- x, ∀x∈R" .Then find fog and gof. Hence find fog(–3), fog(5) and gof (–2).
Which one of the following graphs represents a function?
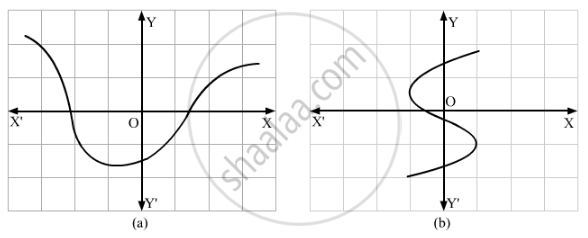
Which of the following graphs represents a one-one function?

If f : C → C is defined by f(x) = (x − 2)3, write f−1 (−1).
If f : R → R is defined by f(x) = 10 x − 7, then write f−1 (x).
\[f : R \to R \text{given by} f\left( x \right) = x + \sqrt{x^2} \text{ is }\]
The function f : R → R defined by
`f (x) = 2^x + 2^(|x|)` is
Which of the following functions form Z to itself are bijections?
Let
\[A = \left\{ x : - 1 \leq x \leq 1 \right\} \text{and} f : A \to \text{A such that f}\left( x \right) = x|x|\]
Which of the following functions from
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\}\]
Let \[f\left( x \right) = x^2 and g\left( x \right) = 2^x\] Then, the solution set of the equation
Let [x] denote the greatest integer less than or equal to x. If \[f\left( x \right) = \sin^{- 1} x, g\left( x \right) = \left[ x^2 \right]\text{ and } h\left( x \right) = 2x, \frac{1}{2} \leq x \leq \frac{1}{\sqrt{2}}\]
If \[f : R \to R\] is given by \[f\left( x \right) = x^3 + 3, \text{then} f^{- 1} \left( x \right)\] is equal to
Let
\[f : R \to R\] be given by \[f\left( x \right) = x^2 - 3\] Then, \[f^{- 1}\] is given by
Mark the correct alternative in the following question:
If the set A contains 7 elements and the set B contains 10 elements, then the number one-one functions from A to B is
Write about strcmp() function.
Let A be a finite set. Then, each injective function from A into itself is not surjective.
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(x, y): x is a person, y is the mother of x}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
g = {(1, 4), (2, 4), (3, 4)}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
h = {(1,4), (2, 5), (3, 5)}
The smallest integer function f(x) = [x] is ____________.
Let f: R→R be a polynomial function satisfying f(x + y) = f(x) + f(y) + 3xy(x + y) –1 ∀ x, y ∈ R and f'(0) = 1, then `lim_(x→∞)(f(2x))/(f(x)` is equal to ______.
Let S = {1, 2, 3, 4, 5, 6, 7}. Then the number of possible functions f: S `rightarrow` S such that f(m.n) = f(m).f(n) for every m, n ∈ S and m.n ∈ S is equal to ______.
Write the domain and range (principle value branch) of the following functions:
f(x) = tan–1 x.
Let A = R – {2} and B = R – {1}. If f: A `→` B is a function defined by f(x) = `(x - 1)/(x - 2)` then show that f is a one-one and an onto function.
