Advertisements
Advertisements
Question
Solution
\[Let f^{- 1} \left( 1 \right) = x . . . \left( 1 \right)\]
\[ \Rightarrow f\left( x \right) = 1\]
\[ \Rightarrow x^4 = 1\]
\[ \Rightarrow x^4 - 1 = 0\]
\[ \Rightarrow \left( x^2 - 1 \right)\left( x^2 + 1 \right) = 0 \left [ \text{using identity}: a^2 - b^2 = \left( a - b \right)\left( a + b \right) \right]\]
\[ \Rightarrow \left( x - 1 \right)\left( x + 1 \right)\left( x^2 + 1 \right) = 0 \left[ \text{using identity}: a^2 - b^2 = \left( a - b \right)\left( a + b \right) \right]\]
\[ \Rightarrow x = \pm 1 \left[ \text{ as } x \in R \right]\]
\[ \Rightarrow f^{- 1} \left( 1 \right) = \left\{ - 1, 1 \right\} [ \text{from}\left( 1 \right)]\]
APPEARS IN
RELATED QUESTIONS
Prove that the greatest integer function f: R → R, given by f(x) = [x], is neither one-one nor onto, where [x] denotes the greatest integer less than or equal to x.
Classify the following function as injection, surjection or bijection : f : N → N given by f(x) = x2
Classify the following function as injection, surjection or bijection : f : Z → Z given by f(x) = x2
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 + 1
Classify the following function as injection, surjection or bijection :
f : Q − {3} → Q, defined by `f (x) = (2x +3)/(x-3)`
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = `x/(x^2 +1)`
If f : R → R be the function defined by f(x) = 4x3 + 7, show that f is a bijection.
If A = {1, 2, 3}, show that a one-one function f : A → A must be onto.
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 2x + x2 and g(x) = x3
Let f = {(1, −1), (4, −2), (9, −3), (16, 4)} and g = {(−1, −2), (−2, −4), (−3, −6), (4, 8)}. Show that gof is defined while fog is not defined. Also, find gof.
Let f : R → R and g : R → R be defined by f(x) = x + 1 and g (x) = x − 1. Show that fog = gof = IR.
Give examples of two functions f : N → Z and g : Z → Z, such that gof is injective but gis not injective.
Let f be a real function given by f (x)=`sqrt (x-2)`
Find each of the following:
(i) fof
(ii) fofof
(iii) (fofof) (38)
(iv) f2
Also, show that fof ≠ `f^2` .
Find f −1 if it exists : f : A → B, where A = {1, 3, 5, 7, 9}; B = {0, 1, 9, 25, 49, 81} and f(x) = x2
Let f : R `{- 4/3} `- 43 →">→ R be a function defined as f(x) = `(4x)/(3x +4)` . Show that f : R - `{-4/3}`→ Rang (f) is one-one and onto. Hence, find f -1.
Let f be a function from R to R, such that f(x) = cos (x + 2). Is f invertible? Justify your answer.
If f : R → R is given by f(x) = x3, write f−1 (1).
If f : R → R is defined by f(x) = 10 x − 7, then write f−1 (x).
Write whether f : R → R, given by `f(x) = x + sqrtx^2` is one-one, many-one, onto or into.
\[f : A \to \text{B given by } 3^{ f\left( x \right)} + 2^{- x} = 4\] is a bijection, then
The function f : [-1/2, 1/2, 1/2] → [-π /2,π/2], defined by f (x) = `sin^-1` (3x - `4x^3`), is
If \[f : R \to R\] is given by \[f\left( x \right) = x^3 + 3, \text{then} f^{- 1} \left( x \right)\] is equal to
Let A = R − (2) and B = R − (1). If f: A ⟶ B is a function defined by`"f(x)"=("x"-1)/("x"-2),` how that f is one-one and onto. Hence, find f−1.
If f(x) = `(x+3)/(4x−5) , "g"(x) = (3+5x)/(4x−1)` then verify that `("fog") (x)` = x.
Show that the function f: R → R defined by f(x) = `x/(x^2 + 1)`, ∀ ∈ + R , is neither one-one nor onto
Let the function f: R → R be defined by f(x) = cosx, ∀ x ∈ R. Show that f is neither one-one nor onto
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
h = {(1,4), (2, 5), (3, 5)}
Let f: R → R be the functions defined by f(x) = x3 + 5. Then f–1(x) is ______.
Let A = R – {3}, B = R – {1}. Let f : A → B be defined by `"f"("x") = ("x" - 2)/("x" - 3)` Then, ____________.
Let f : R `->` R be a function defined by f(x) = x3 + 4, then f is ______.
Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1,2,3,4,5,6}. Let A be the set of players while B be the set of all possible outcomes.
A = {S, D}, B = {1,2,3,4,5,6}
- Raji wants to know the number of functions from A to B. How many number of functions are possible?
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Ravi wants to find the number of injective functions from B to G. How many numbers of injective functions are possible?
If f: R → R given by f(x) =(3 − x3)1/3, find f0f(x)
If `f : R -> R^+ U {0}` be defined by `f(x) = x^2, x ∈ R`. The mapping is
'If 'f' is a linear function satisfying f[x + f(x)] = x + f(x), then f(5) can be equal to:
If A = {x ∈ R: |x – 2| > 1}, B = `{x ∈ R : sqrt(x^2 - 3) > 1}`, C = {x ∈ R : |x – 4| ≥ 2} and Z is the set of all integers, then the number of subsets of the set (A ∩ B ∩ C) C ∩ Z is ______.
Write the domain and range (principle value branch) of the following functions:
f(x) = tan–1 x.
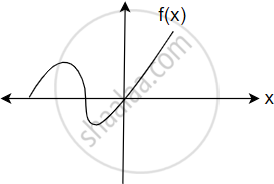
The given function f : R → R is not ‘onto’ function. Give reason.
