Advertisements
Advertisements
प्रश्न
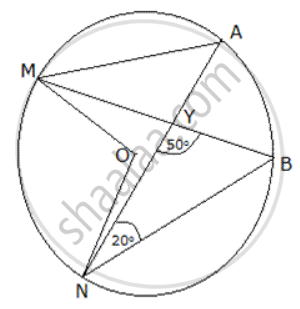
MABN are points on a drde having centre O. AN and MB cut at Y. If ∠ NYB = 50" and ∠ YNB = 200, find ∠ MAN and reflex angle MON.
उत्तर
∠ NYB =50 °
∠ YNB = 20°
In Δ NYB,
20° + ∠ NBY + 50 ° = 180°
⇒ ∠ NBY = 180° - 70 ° = 110 °
Now, ∠ MAN = ∠ NBM = 110° (Angles in the same segment)
∠ MON = 2 ∠ MAN (Arc MN subtends ∠ MON at centre and ∠ MAN at remaining part of the circle)
∠ MON = 2(110°) = 220°
Reflex ∠ MON = 360° - ∠ MON
= 360° - 220°
Reflex ∠ MON = 140°.
APPEARS IN
संबंधित प्रश्न
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :
- angle BCT
- angle DOC

In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.

If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
- EF = FC
- BF = DF
In a cyclic quadrialteral ABCD , if m ∠ A = 3 (m ∠C). Find m ∠ A.
In a circle with centre O , chords AB and CD intersets inside the circle at E . Prove that ∠ AOC = ∠ BOD = 2 ∠ AEC.
Prove that the angles bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (provided they are not parallel) intersect at right triangle.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.
If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.
The diagonals of a cyclic quadrilateral are at right angles. Prove that the perpendicular from the point of their intersection on any side when produced backward bisects the opposite side.
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.
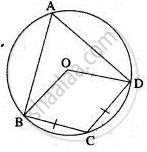
In the given figure, the sides of the quadrilateral PQRS touches the circle at A, B, C and D. If RC = 4 cm, RQ = 7 cm and PD = 5 cm. Find the length of PQ: