Advertisements
Advertisements
प्रश्न
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :
- angle BCT
- angle DOC

उत्तर
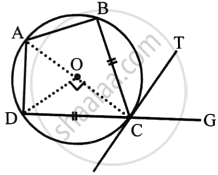
Join OC, OD and AC
i. ∠BCG + ∠BCD = 180° ...(Linear pair)
`=>` 108° + ∠BCD = 180° ...(∵ ∠BCG = 108° given)
`=>` ∠BCD = 180° – 108° = 72°
BC = CD ...(Given)
∴ ∠DCP = ∠BCT
But, ∠BCT + ∠BCD + ∠DCP = 180°
∴ ∠BCT + ∠BCT + 72° = 180° ...(∵ ∠DCP = ∠BCT)
2∠BCT = 180° – 72° = 108°
∴ ∠BCT = `108^circ/2` = 54°
ii. PCT is a tangent and CA is a chord.
∠CAD = ∠BCT = 54°
But arc DC subtends ∠DOC at the centre and ∠CAD at the remaining part of the circle.
∴ ∠DOC = 2∠CAD
= 2 × 54°
= 108°
APPEARS IN
संबंधित प्रश्न
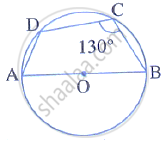
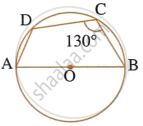
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

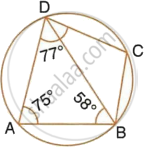
In the figure, given below, ABCD is a cyclic quadrilateral in which ∠BAD = 75°; ∠ABD = 58° and ∠ADC = 77°. Find:
- ∠BDC,
- ∠BCD,
- ∠BCA.
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130°. Find:
(i) ∠DAB
(ii) ∠DBA
D and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD = AE. Prove that the points B, C, E and D are concyclic.
In the following figure, O is the centre of the circle. Find the values of a, b and c.

If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.
If O is the centre of the circle, find the value of x in each of the following figures

In the given figure, AB is the diameter of a circle with centre O.
∠BCD = 130°. Find:
- ∠DAB
- ∠DBA
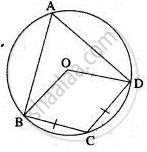
In the given figure O is the center of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
(i) ∠BOC (ii) ∠OBD (iii) ∠BCD.
An exterior angle of a cyclic quadrilateral is congruent to the angle opposite to its adjacent interior angle, to prove the theorem complete the activity.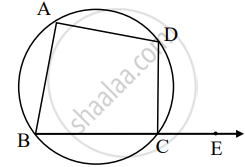
Given: ABCD is cyclic,
`square` is the exterior angle of ABCD
To prove: ∠DCE ≅ ∠BAD
Proof: `square` + ∠BCD = `square` .....[Angles in linear pair] (I)
ABCD is a cyclic.
`square` + ∠BAD = `square` ......[Theorem of cyclic quadrilateral] (II)
By (I) and (II)
∠DCE + ∠BCD = `square` + ∠BAD
∠DCE ≅ ∠BAD
