Advertisements
Advertisements
प्रश्न
Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals
उत्तर
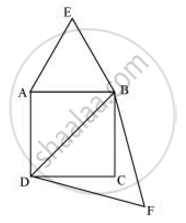
Let ABCD be a square of side a.
Therefore, its diagonal = `sqrt2a`
Two desired equilateral triangles are formed as ΔABE and ΔDBF.
Side of an equilateral triangle, ΔABE, described on one of its sides = a
Side of an equilateral triangle, ΔDBF, described on one of its diagonals = `sqrt2a`
We know that equilateral triangles have all its angles as 60º and all its sides of the same length. Therefore, all equilateral triangles are similar to each other. Hence, the ratio between the areas of these triangles will be equal to the square of the ratio between the sides of these triangles.
`"Area of Δ ABE"/"Area of ΔDBF"=(a/(sqrt2a))^2`
`"Area of Δ ABE"/"Area of ΔDBF"=(1/sqrt2)^2=1/2`
APPEARS IN
संबंधित प्रश्न
If ∆ABC is similar to ∆DEF such that ∆DEF = 64 cm2 , DE = 5.1 cm and area of ∆ABC = 9 cm2 . Determine the area of AB
If ∆ABC ~ ∆DEF such that area of ∆ABC is 16cm2 and the area of ∆DEF is 25cm2 and BC = 2.3 cm. Find the length of EF.
D, E, F are the mid-point of the sides BC, CA and AB respectively of a ∆ABC. Determine the ratio of the areas of ∆DEF and ∆ABC.
D and E are points on the sides AB and AC respectively of a ∆ABC such that DE || BC and divides ∆ABC into two parts, equal in area. Find
Two isosceles triangles have equal vertical angles and their areas are in the ratio 16 : 25. Find the ratio of their corresponding heights
If the areas of two similar triangles are equal, prove that they are congruent.
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
In Figure, DE || BC If DE = 4 cm, BC = 6 cm and Area (ΔADE) = 16 cm2, find the area of ΔABC.

If ΔABC is similar to ΔDEF such that 2 AB = DE and BC = 8 cm then EF is equal to ______.
If ΔABC ∼ ΔPQR and `(A(ΔABC))/(A(ΔPQR)) = 16/25`, then find AB : PQ.
