Advertisements
Advertisements
प्रश्न
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
उत्तर
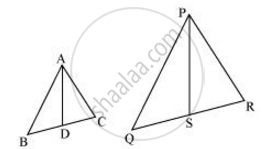
Let us assume two similar triangles as ΔABC ∼ ΔPQR. Let AD and PS be the medians of these triangles.
∵ ΔABC ∼ ΔPQR
:.(AB)/(PQ) = (BC)/(QR) = (AC)/(PR)...(1)
∠A = ∠P, ∠B = ∠Q, ∠C = ∠R … (2)
Since AD and PS are medians,
∴ BD = DC = `(BC)/2`
And, QS = SR = `(QR)/2`
Equation (1) becomes
(AB)/(PQ) = (BD)/(QS) = (AC)/(PR) ....(3)
In ΔABD and ΔPQS,
∠B = ∠Q [Using equation (2)]
and (AB)/(PQ) = (BD)/(QS) [Using equation (3)]
∴ ΔABD ∼ ΔPQS (SAS similarity criterion)
Therefore, it can be said that
`(AB)/(PQ) = (BD)/(QS) =(AD)/(PS) ....(4)`
`(ar(triangleABC))/(ar(trianglePQR)) = ((AB)/(PQ))^2 = ((BC)/(QR))^2 = ((AC)/(PR))^2`
From equations (1) and (4), we may find that
`(AB)/(PQ) = (BC)/(QR) = (AC)/(PR) = (AD)/(PS)`
And hence
`(ar(triangleABC))/(ar(trianglePQR)) = ((AD)/(PS))^2`
APPEARS IN
संबंधित प्रश्न
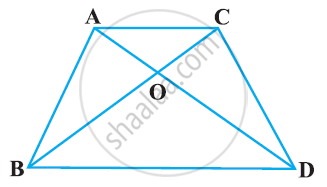
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
If the areas of two similar triangles are equal, prove that they are congruent.
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.
In Figure, DE || BC If DE = 4 cm, BC = 6 cm and Area (ΔADE) = 16 cm2, find the area of ΔABC.

If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
In the given figure 1.66, seg PQ || seg DE, A(∆PQF) = 20 units, PF = 2 DP, then Find A(◻DPQE) by completing the following activity.

∆MNT ~ ∆QRS. Length of altitude drawn from point T is 5 and length of altitude drawn from point S is 9. Find the ratio `("A"(Δ"MNT"))/("A"(Δ"QRS"))`.
The perpendicular from A on side BC of a Δ ABC meets BC at D such that DB = 3CD. Prove that 2AB2 = 2AC2 + BC2.
Prove that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
If ΔABC is similar to ΔDEF such that 2 AB = DE and BC = 8 cm then EF is equal to ______.
