Advertisements
Advertisements
प्रश्न
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
उत्तर
Given:
∆ABC ~ ∆PQR
A (∆ABC) = 80
A (∆PQR) = 125
According to theorem of areas of similar triangles "When two triangles are similar, the ratio of areas of those triangles is equal to the ratio of the squares of their corresponding sides".
\[\therefore \frac{A\left( ∆ ABC \right)}{A\left( ∆ PQR \right)} = \frac{{AB}^2}{{PQ}^2}\]
\[ \Rightarrow \frac{80}{125} = \frac{{AB}^2}{{PQ}^2}\]
\[ \Rightarrow \frac{16}{25} = \frac{{AB}^2}{{PQ}^2}\]
\[\Rightarrow \frac{4^2}{5^2} = \frac{{AB}^2}{{PQ}^2}\]
\[ \Rightarrow \frac{AB}{PQ} = \frac{4}{5}\]
Therefore,
\[\frac{A\left( ∆ ABC \right)}{A\left( ∆ PQR \right)} = \frac{80}{125} and \frac{AB}{PQ} = \frac{4}{5}\]
संबंधित प्रश्न
In a trapezium ABCD, O is the point of intersection of AC and BD, AB || CD and AB = 2 × CD. If the area of ∆AOB = 84 cm2 . Find the area of ∆COD
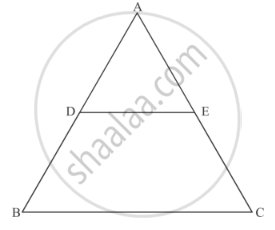
D and E are points on the sides AB and AC respectively of a ∆ABC such that DE || BC and divides ∆ABC into two parts, equal in area. Find
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2 CD, find the ratio of the areas of triangles AOB and COD.
If the areas of two similar triangles are equal, prove that they are congruent.
D, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the area of ΔDEF and ΔABC.
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
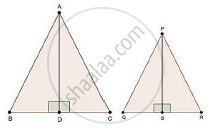
The corresponding altitudes of two similar triangles are 6 cm and 9 cm respectively. Find the ratio of their areas.
In Figure, DE || BC If DE = 4cm, BC = 8 cm and Area (ΔADE) = 25 cm2, find the area of ΔABC.
ABCD is a trapezium in which AB || CD. The diagonals AC and BD intersect at O. Prove that: (i) ΔAOB and ΔCOD (ii) If OA = 6 cm, OC = 8 cm,
Find:(a) `("area"(triangleAOB))/("area"(triangleCOD))`
(b) `("area"(triangleAOD))/("area"(triangleCOD))`
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 m, prove that area of ΔAPQ is one- sixteenth of the area of ABC.
Ratio of areas of two similar triangles is 9 : 25. _______ is the ratio of their corresponding sides.
In the given figure, ΔACB ~ ΔAPQ. If AB = 6 cm, BC = 8 cm, and PQ = 4 cm then AQ is equal to ______.

In ΔLMN, ∠L = 50° and ∠N = 60°, If ΔLMN ~ ΔPQR, then find ∠Q.
In a rectangle Length = 8 cm, Breadth = 6 cm. Then its diagonal = ______.
Find the length of ST, if ΔPQR ∼ ΔPST.

In ΔABC, seg DE || side BC. If 2A(ΔADE) = A(⬜ DBCE), find AB : AD and show that BC = `sqrt(3)` DE.
If ΔABC ∼ ∆PQR and AB : PQ = 2 : 3, then find the value of `(A(triangleABC))/(A(trianglePQR))`.
