Advertisements
Advertisements
प्रश्न
In ΔABC, seg DE || side BC. If 2A(ΔADE) = A(⬜ DBCE), find AB : AD and show that BC = `sqrt(3)` DE.
उत्तर
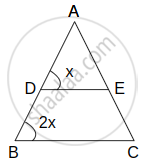
∴ Given: In `triangleABC`, DE || side BC.
`2A(triangleADE) = A(triangleDBCE)`
To find: `(AB)/(AD)`
To prove: BC = `sqrt3DE`
Proof: In `triangle ABC`
DE | | BC
∴ ∠ADE = ∠ ABC ...Corresponding angle ... (i)
∴ In `triangleADE` and `triangleABC` ∠BAC = ∠DAE ...Common angle
∠ADE = ∠ABC ...By (i)
∴ By A - A test
`triangleADE ≅ triangleABC`
`(AB)/(AD) = (BC)/(DE) = (AC)/(AE)`
Also, `2A(triangleADE) = A(DBCE)`
as `A(triangleABC) = A(triangleADE) +2(DBCE)`
`A(triangleABC) = A(triangleADE) + 2A(triangleADE)`
`A(triangleABC) = 3A(triangleADE)`
`(A(triangleABC))/(A(triangleADE) )= 3/1` ...(2)
`(A(triangleABC))/(A(triangleADE) )= (BC^2)/(DE^2)`
`3/1 = (BC^2)/(DE^2)` ...(Theorem of Area of similar triangle)
`3DE^2 = BC^2`
`sqrt3DE = BC` ...(By taking square root on both sides)
`BC = sqrt3 DE` Hence proved
APPEARS IN
संबंधित प्रश्न
D and E are points on the sides AB and AC respectively of a ∆ABC such that DE || BC and divides ∆ABC into two parts, equal in area. Find
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, find the longest side of the smaller triangle.
In Figure, DE || BC If DE = 4 cm, BC = 6 cm and Area (ΔADE) = 16 cm2, find the area of ΔABC.

In ΔABC, D and E are the mid-points of AB and AC respectively. Find the ratio of the areas of ΔADE and ΔABC
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divides ΔABC into two parts equal in area. Find `(BP)/(AB)`
The areas of two similar triangles ABC and PQR are in the ratio 9:16. If BC = 4.5 cm, find the length of QR.
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
∆LMN ~ ∆PQR, 9 × A (∆PQR ) = 16 × A (∆LMN). If QR = 20 then Find MN.
ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
The perpendicular from A on side BC of a Δ ABC meets BC at D such that DB = 3CD. Prove that 2AB2 = 2AC2 + BC2.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that seg PQ || seg BC. If PQ divides ΔABC into two equal parts having equal areas, find `"BP"/"AB"`.
If ∆XYZ ~ ∆PQR and A(∆XYZ) = 25 cm2, A(∆PQR) = 4 cm2 then XY : PQ = ?
Ratio of areas of two similar triangles is 9 : 25. _______ is the ratio of their corresponding sides.
In a rhombus if d1 = 16 cm, d2 = 12 cm, its area will be ______.
In the adjoining figure, ΔADB ∼ ΔBDC. Prove that BD2 = AD × DC.
If ΔPQR ∼ ΔABC; PQ = 6 cm, AB = 8 cm and the perimeter of ΔABC is 36 cm, then the perimeter of ΔPQR is ______.
