Advertisements
Advertisements
Question
Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals
Solution
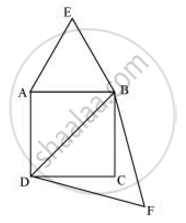
Let ABCD be a square of side a.
Therefore, its diagonal = `sqrt2a`
Two desired equilateral triangles are formed as ΔABE and ΔDBF.
Side of an equilateral triangle, ΔABE, described on one of its sides = a
Side of an equilateral triangle, ΔDBF, described on one of its diagonals = `sqrt2a`
We know that equilateral triangles have all its angles as 60º and all its sides of the same length. Therefore, all equilateral triangles are similar to each other. Hence, the ratio between the areas of these triangles will be equal to the square of the ratio between the sides of these triangles.
`"Area of Δ ABE"/"Area of ΔDBF"=(a/(sqrt2a))^2`
`"Area of Δ ABE"/"Area of ΔDBF"=(1/sqrt2)^2=1/2`
APPEARS IN
RELATED QUESTIONS
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
If the areas of two similar triangles are equal, prove that they are congruent.
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the area of triangles ABC and BDE is
ABC is a triangle in which ∠A =90°, AN⊥ BC, BC = 12 cm and AC = 5cm. Find the ratio of the areas of ΔANC and ΔABC.
If ΔABC ~ ΔDEF such that AB = 5 cm, area (ΔABC) = 20 cm2 and area (ΔDEF) = 45 cm2, determine DE.
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 m, prove that area of ΔAPQ is one- sixteenth of the area of ABC.
If ∆ABC ~ ∆PQR and AB : PQ = 2 : 3, then fill in the blanks.
\[\frac{A\left( ∆ ABC \right)}{A\left( ∆ PQR \right)} = \frac{{AB}^2}{......} = \frac{2^2}{3^2} = \frac{......}{.......}\]
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that seg PQ || seg BC. If PQ divides ΔABC into two equal parts having equal areas, find `"BP"/"AB"`.
Ratio of areas of two similar triangles is 9 : 25. _______ is the ratio of their corresponding sides.
In a rhombus if d1 = 16 cm, d2 = 12 cm, its area will be ______.
