Advertisements
Advertisements
Question
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the area of triangles ABC and BDE is
Options
2 : 1
1 : 2
4 : 1
1 : 4
Solution
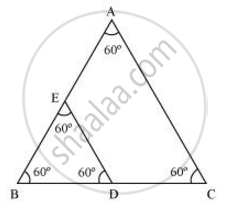
We know that equilateral triangles have all its angles as 60º and all its sides of the same length. Therefore, all equilateral triangles are similar to each other. Hence, the ratio between the areas of these triangles will be equal to the square of the ratio between the sides of these triangles.
Let side of ΔABC = x
Therefore, side of ΔBDE = `x/2`
`∴ (area(ΔABC))/(area(ΔBDE)) = (x/(x/2))^2 =4/1`
Hence, the correct answer is (C).
APPEARS IN
RELATED QUESTIONS
The areas of two similar triangles ∆ABC and ∆PQR are 25 cm2 and 49 cm2 respectively. If QR = 9.8 cm, find BC
D, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the area of ΔDEF and ΔABC.
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.
The areas of two similar triangles are 100 cm2 and 49 cm2 respectively. If the altitude the bigger triangle is 5 cm, find the corresponding altitude of the other.
The ratio of corresponding sides of similar triangles is 3 : 5; then find the ratio of their areas.
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
The perpendicular from A on side BC of a Δ ABC meets BC at D such that DB = 3CD. Prove that 2AB2 = 2AC2 + BC2.
In the given figure DE || AC which of the following is true?
In ΔLMN, ∠L = 50° and ∠N = 60°, If ΔLMN ~ ΔPQR, then find ∠Q.
In a rectangle Length = 8 cm, Breadth = 6 cm. Then its diagonal = ______.
