Advertisements
Advertisements
प्रश्न
Show that the rectangle of the maximum perimeter which can be inscribed in the circle of radius 10 cm is a square of side `10sqrt2` cm.
उत्तर
`AB = 2 xx r cos theta`, `AD = 2r sin theta`
here r = 10
`AB = 20 cos theta, AD = 20 sin theta`
`A = 2rcos theta.2r sin theta` {Here r = 10}
`A= 4r^2 cos theta.sin theta`
`= 2r^2. 2sin theta cos theta`
`= 2 xx 100 sin 2 theta`
`(dA)/(d theta) = 200 cos 2 theta . 2`
`(dA)/(d theta) = 0`
`=> cos 2 theta = 0`
`2 theta = pi/2`
`theta = pi/4`
`(dA^2)/(d theta^2) = 400 (-2 sin 2 theta)`
`(dA^2)/(d theta^2) = -800 sin pi/2 = - 800< 0`
So, Area is max, when `AB = 20 cos pi/4 = 10sqrt2`
`AD = 20 cos pi/4 = 10sqrt2`
`:. AB = AD = 10sqrt2`
APPEARS IN
संबंधित प्रश्न
Find the area of the circle 4x2 + 4y2 = 9 which is interior to the parabola x2 = 4y
Find the area of the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 3
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
A. 2 (π – 2)
B. π – 2
C. 2π – 1
D. 2 (π + 2)
Area lying between the curve y2 = 4x and y = 2x is
A. 2/3
B. 1/3
C. 1/4
D. 3/4
Find the area bounded by curves {(x, y) : y ≥ x2 and y = |x|}.
Choose the correct answer The area of the circle x2 + y2 = 16 exterior to the parabola y2 = 6x is
A. `4/3 (4pi - sqrt3)`
B. `4/3 (4pi + sqrt3)`
C. `4/3 (8pi - sqrt3)`
D.`4/3 (4pi + sqrt3)`
The area bounded by the y-axis, y = cos x and y = sin x when 0 <= x <= `pi/2`
(A) 2 ( 2 −1)
(B) `sqrt2 -1`
(C) `sqrt2 + 1`
D. `sqrt2`
Find the area included between the parabolas y2 = 4ax and x2 = 4by.
The area enclosed between the curves y = loge (x + e), x = loge \[\left( \frac{1}{y} \right)\] and the x-axis is _______ .
The area between x-axis and curve y = cos x when 0 ≤ x ≤ 2 π is ___________ .
Area enclosed between the curve y2 (2a − x) = x3 and the line x = 2a above x-axis is ___________ .
Area lying between the curves y2 = 4x and y = 2x is
Solve the following :
Find the area of the region lying between the parabolas :
y2 = 4x and x2 = 4y
The area enclosed between the two parabolas y2 = 20x and y = 2x is ______ sq.units
Find the area enclosed between the X-axis and the curve y = sin x for values of x between 0 to 2π
Find the area of the ellipse `x^2/36 + y^2/64` = 1, using integration
Find the area of the region included between y = x2 + 5 and the line y = x + 7
Find the area of the region included between the parabola y = `(3x^2)/4` and the line 3x – 2y + 12 = 0.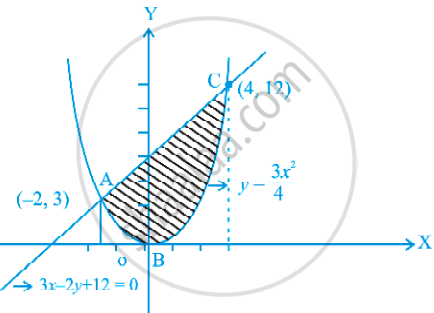
Find the area of the region bounded by the curves x = at2 and y = 2at between the ordinate corresponding to t = 1 and t = 2.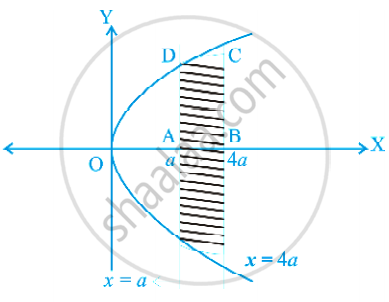
Determine the area under the curve y = `sqrt("a"^2 - x^2)` included between the lines x = 0 and x = a.
Let the area enclosed by the x-axis, and the tangent and normal drawn to the curve 4x3 – 3xy2 + 6x2 – 5xy – 8y2 + 9x + 14 = 0 at the point (–2, 3) be A. Then 8A is equal to ______.
Find the area enclosed between 3y = x2, X-axis and x = 2 to x = 3.
Find the area cut off from the parabola 4y = 3x2 by the line 2y = 3x + 12.
