Advertisements
Advertisements
प्रश्न
The equation of the line passing through (1, 2) and perpendicular to x + y + 7 = 0 is ______.
पर्याय
y – x + 1 = 0
y – x – 1 = 0
y – x + 2 = 0
y – x – 2 = 0.
उत्तर
The equation of the line passing through (1, 2) and perpendicular to x + y + 7 = 0 is y – x – 1 = 0.
Explanation:
. Let the slope of the line be m.
Then, its equation passing through (1, 2) is given by
y – 2 = m(x – 1) ....(1)
Again, this line is perpendicular to the given line x + y + 7 = 0 whose slope is – 1 (Why?)
Therefore, we have m ( – 1) = – 1
or m = 1
Hence, the required equation of the line is obtained by putting the value of m in (1)
i.e., y – 2 = x – 1
or y – x – 1 = 0
APPEARS IN
संबंधित प्रश्न
The base of an equilateral triangle with side 2a lies along they y-axis such that the mid point of the base is at the origin. Find vertices of the triangle.
Without using distance formula, show that points (–2, –1), (4, 0), (3, 3) and (–3, 2) are vertices of a parallelogram.
The slope of a line is double of the slope of another line. If tangent of the angle between them is `1/3`, find the slopes of the lines.
Find the values of k for which the line (k–3) x – (4 – k2) y + k2 –7k + 6 = 0 is
- Parallel to the x-axis,
- Parallel to the y-axis,
- Passing through the origin.
Find the equation of a line drawn perpendicular to the line `x/4 + y/6 = 1`through the point, where it meets the y-axis.
Find the slope of the lines which make the following angle with the positive direction of x-axis:
\[\frac{3\pi}{4}\]
Using the method of slope, show that the following points are collinear A (16, − 18), B (3, −6), C (−10, 6) .
What is the value of y so that the line through (3, y) and (2, 7) is parallel to the line through (−1, 4) and (0, 6)?
The slope of a line is double of the slope of another line. If tangents of the angle between them is \[\frac{1}{3}\],find the slopes of the other line.
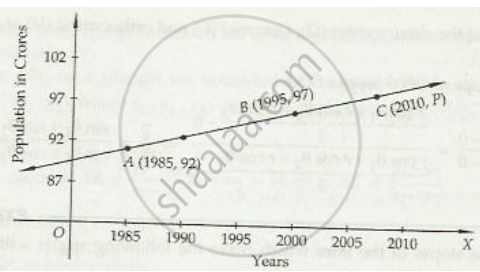
Consider the following population and year graph:
Find the slope of the line AB and using it, find what will be the population in the year 2010.
Line through the points (−2, 6) and (4, 8) is perpendicular to the line through the points (8, 12) and (x, 24). Find the value of x.
A quadrilateral has vertices (4, 1), (1, 7), (−6, 0) and (−1, −9). Show that the mid-points of the sides of this quadrilateral form a parallelogram.
Find the equation of a straight line with slope 2 and y-intercept 3 .
Find the equation of a straight line with slope −2 and intersecting the x-axis at a distance of 3 units to the left of origin.
Find the equations of the bisectors of the angles between the coordinate axes.
Find the equation of the strainght line intersecting y-axis at a distance of 2 units above the origin and making an angle of 30° with the positive direction of the x-axis.
Prove that the points (2, −1), (0, 2), (2, 3) and (4, 0) are the coordinates of the vertices of a parallelogram and find the angle between its diagonals.
Write the coordinates of the image of the point (3, 8) in the line x + 3y − 7 = 0.
The equation of the line with slope −3/2 and which is concurrent with the lines 4x + 3y − 7 = 0 and 8x + 5y − 1 = 0 is
If the slope of a line passing through the point A(3, 2) is `3/4`, then find points on the line which are 5 units away from the point A.
The two lines ax + by = c and a′x + b′y = c′ are perpendicular if ______.
Find the angle between the lines y = `(2 - sqrt(3)) (x + 5)` and y = `(2 + sqrt(3))(x - 7)`
Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.
A variable line passes through a fixed point P. The algebraic sum of the perpendiculars drawn from the points (2, 0), (0, 2) and (1, 1) on the line is zero. Find the coordinates of the point P.
The tangent of angle between the lines whose intercepts on the axes are a, – b and b, – a, respectively, is ______.
Equation of the line passing through (1, 2) and parallel to the line y = 3x – 1 is ______.
The vertex of an equilateral triangle is (2, 3) and the equation of the opposite side is x + y = 2. Then the other two sides are y – 3 = `(2 +- sqrt(3)) (x - 2)`.
The three straight lines ax + by = c, bx + cy = a and cx + ay = b are collinear, if ______.
