Advertisements
Advertisements
प्रश्न
The length and breadth of a rectangular garden are in the ratio 9:5. A path 3.5 m wide, running all around inside it has an area of `1911m^2` . Find the dimensions of the garden.
उत्तर
Let the length and breadth of the garden be 9x m and 5x m, respectively, Now,
Area of the garden=`(9x xx 5x)45x^2`
Length of the garden excluding the path =`(9x-7)`
Breadth of the garden excluding the path =`(5x-7)`
Area of the path = `45x^2=[(9x-7)(5x-7)]`
⇒ `1911=45x^2-[45x^2-63x-35x+49]`
⇒`1911=45x^2-45x^2+63x+35x-49`
⇒`1911=98x-49`
⇒`1960=98x`
⇒`x=1960/98`
⇒ `x=20`
Thus, we have:
Length=`9x=20xx9=180m`
Breadth=`5x=5xx20=100m`
APPEARS IN
संबंधित प्रश्न
A square of diagonal 8 cm is inscribed in a circle. Find the area of the region lying outside the circle and inside the square.
A circular field has a perimeter of 650 m. A square plot having its vertices on the circumference of the field is marked in the field. Calculate the area of the square plot.
If the circumference of two circles are in the ratio 2 : 3, what is the ratio of their areas?
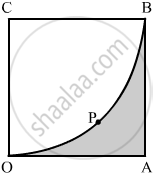
In the given figure, OABC is a square of side 7 cm. If COPB is a quadrant of a circle with centre C find the area of the shaded region.
Find the area of a ring whose outer and inner radii are respectively 23 cm and 12 cm.
The area of circle is equal to the sum of the areas of two circles of radii 24 cm and 7 cm. The diameter of the new circle is
The radius of a wheel is 0.25 m. How many revolutions will it make in covering 11 km?
A chord of a circle of radius 14 cm a makes a right angle at the centre. Find the area of the sector.
The given figures show a rectangle ABCD inscribed in a circle as shown alongside.
If AB = 28 cm and BC = 21 cm, find the area of the shaded portion of the given figure.
Find the area of a ring-shaped region enclosed between two concentric circles of radii 20 cm and 15 cm.
