Question

The lower window of a house is at a height of 2 m above the ground and its upper window is 4 m vertically above the lower window. At certain instant the angles of elevation of a balloon from these windows are observed to be 60° and 30° respectively. Find the height of the balloon above the ground.

Answer 1

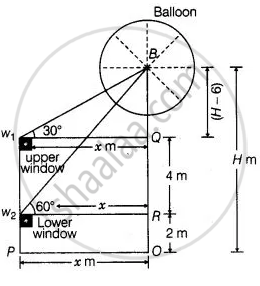
Let the height of the balloon from above the ground is H.

A and OP = w₂R = w₁Q = x

Given that, height of lower window from above the ground = w₂P = 2 m = OR

Height of upper window from above the lower window = w₁w₂ = 4 m = QR

∴ BQ = OB – (QR + RO)

= H – (4 + 2)

= H – 6

And ∠Bw₁Q = 30°

⇒ ∠Bw₂R = 60°

Now, in ΔBw₂R,

tan 60° = `"BR"/("w"_2"R") = ("BQ" + "QR")/x`

⇒ `sqrt(3) = (("H" - 6) + 4)/x`

⇒ `x = ("H" - 2)/sqrt(3)`  ...(i)

And in ΔBw₁Q,

tan 30° = `"BQ"/("w"_1"Q")`

tan 30° = `("H" - 6)/x = 1/sqrt(3)`

⇒ `x = sqrt(3)("H" - 6)`  ...(ii)

From equations (i) and (ii),

`sqrt(3)("H" - 6) = (("H" - 2))/sqrt(3)`

⇒ 3(H – 6) = H – 2

⇒ 3H – 18 = H – 2

⇒ 2H = 16

⇒ H = 8

So, the required height is 8 m.

Hence, the required height of the balloon from above the ground is 8 m.