Advertisements
Advertisements
प्रश्न
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. Find the coordinates of the point P on AD such that AP : PD = 2 : 1
उत्तर
According to the question,
The vertices of ΔABC = A, B and C
Coordinates of A, B and C = A(x1, y1), B(x2, y2), C(x3, y3)
Let the coordinates of a point P be (x, y)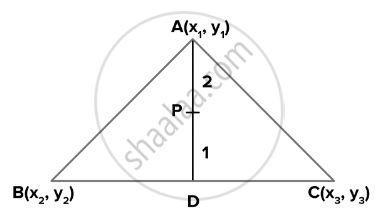
Given,
The ratio in which the point P(x, y), divide the line joining,
`"A"(x_1, y_1)` and `"D"((x_2 + x_3)/2, (y_2 + y_3)/2)` = 2 : 1
Then,
Coordinates of P = `[(2 xx ((x_2 + x_3)/2) + 1 xx x_1)/(2 + 1), (2 xx ((y_2 + y_3)/2) + 1 xx y_1)/(2 + 1)]`
By using internal section formula;
= `((m_1x_2 + m_2x_1)/(m_1 + m_2), (m_1y_2 + m_2y_1)/(m_1 + m_2))`
= `((x_2 + x_3 + x_1)/3, (y_2 + y_3 + y_1)/3)`
APPEARS IN
संबंधित प्रश्न
Find the coordinates of the point which divides the join of (–1, 7) and (4, –3) in the ratio 2 : 3.
Find the ratio in which the line segment joining A (1, −5) and B (−4, 5) is divided by the x-axis. Also, find the coordinates of the point of division.
In what ratio does the point `(24/11, y)` divide the line segment joining the points P(2, –2) and Q(3, 7)? Also find the value of y.
Find the lengths of the medians of a triangle whose vertices are A (−1,3), B(1,−1) and C(5, 1).
Find the ratio in which the join of (–4, 7) and (3, 0) is divided by the y-axis. Also, find the co-ordinates of the point of intersection.
Points A, B, C and D divide the line segment joining the point (5, –10) and the origin in five equal parts. Find the co-ordinates of B and D.
The line joining the points A (–3, –10) and B (–2, 6) is divided by the point P such that `(PB)/(AB) = 1/5`. Find the co-ordinates of P.
Find the coordinates of a point P, which lies on the line segment joining the points A (−2, −2), and B (2, −4), such that `AP=3/7 AB`.
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
Show that the lines x = O and y = O trisect the line segment formed by joining the points (-10, -4) and (5, 8). Find the points of trisection.
