Advertisements
Advertisements
प्रश्न
Two boats approach a lighthouse in mid-sea from opposite directions. The angles of elevation of the top of the lighthouse from two boats are 30° and 45° respectively. If the distance between two boats is 100 m, find the height of the lighthouse.
उत्तर
Let h be the height of lighthouse BD. An angle of elevation of the top of the lighthouse from two boats is 30° and 45°.
Let DB = h , BC = x and it is given that AC = 100 m. So
AB = 100 - x. And ∠DAB = 30°, ∠BCD = 45°
Here we have to find height of light house.
The corresponding figure is as follows
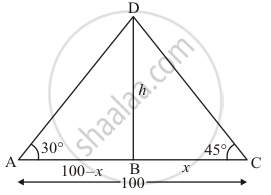
So we use trigonometric ratios.
In ΔBDC
`=> tan 45° = (BD)/(BC)`
`=> 1 = h/x`
`=> x = h `
Again in ΔDAB
`=> tan 30° = (DB)/(AB)`
`=> 1/sqrt3 = h/(100 - x)`
`=> sqrt3h = 100 - x`
`=> (sqrt3 + 1)h = 100`
`=> h = 100/(sqrt3 + 1) xx (sqrt3 - 1)/(sqrt3 - 1)`
`=> h = 50(sqrt3 - 1)`
Hence the height of light house is `50(sqrt3 - 1)` m
APPEARS IN
संबंधित प्रश्न
At a point A, 20 metres above the level of water in a lake, the angle of elevation of a cloud is 30˚. The angle of depression of the reflection of the cloud in the lake, at A is 60˚.
Find the distance of the cloud from A.
An aeroplane flying horizontally 1 km above the ground is observed at an elevation of 60°. After 10 seconds, its elevation is observed to be 30°. Find the speed of the aeroplane in km/hr.
The angles of elevation of the top of a rock from the top and foot of a 100 m high tower are respectively 30° and 45°. Find the height of the rock.
On a horizonal plane there is a vertical tower with a flagpole on the top of the tower. At a point, 9 meters away from the foot of the tower, the angle of elevation of the top and bottom of the flagpole are 60 and 30 respectively. Find the height of the tower and the flagpole mounted on it.
Two poles of heights 18 metre and 7 metre are erected on a ground. The length of the wire fastened at their tops in 22 metre. Find the angle made by the wire with the horizontal.
If the altitude of the sum is at 60°, then the height of the vertical tower that will cast a shadow of length 30 m is
The angle of elevation of a cloud from a point h metre above a lake is θ. The angle of depression of its reflection in the lake is 45°. The height of the cloud is
The electric pole subtends an angle of 30° at a point on the same level as its foot. At a second point ‘b’ metres above the first, the depression of the foot of the pole is 60°. The height of the pole (in metres) is equal to
A Technician has to repair light on a pole of height 10 m. She needs to reach a point 1 m below the top of the pole to undertake the repair work. What should be the length of the ladder that she should use which, when inclined at an angle of 60∘ to the ground, would enable her to reach the required position? Also, how far from the foot of the pole should she place the foot of the ladder?
A TV tower stands vertically on a bank of a canal, with a height of `10 sqrt3` m. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From another point on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30°. Find the distance between the opposite bank of the canal and the point with a 30° angle of elevation.
