Advertisements
Advertisements
प्रश्न
Two cones with same base radius 8 cm and height 15 cm are joined together along their bases. Find the surface area of the shape so formed.
उत्तर
According to the question,
We get the figure given below,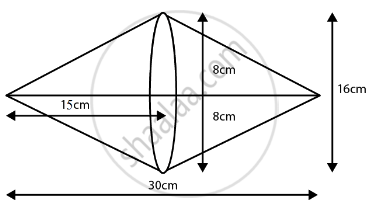
We know that,
Total surface area of shape formed = Curved area of first cone + Curved surface area of second cone
Since, both cones are identical,
We have,
Total surface area of shape formed = Curved area of first cone + Curved surface area of the second cone
= 2(Surface area of cone)
We also know that,
Surface area of cone = πrl, where r = radius and l = slant height
And the total surface area of shape so formed = 2πrl
Given in the question that,
Radius, r = 8 cm
Height, h = 15 cm
Therefore,
Area = Curved area of first cone + Curved surface area of the second cone
= 2(Surface area of the cone)
= 2 × πrl
= `2 xx π xx "r" xx sqrt("r"^2 + "h"^2)`
= `2 xx 22/7 xx 8 xx sqrt(8^2 + 15^2)`
= `50.28 xx sqrt(289)`
= 854.85 cm2
= 855 cm2 ...(Approx)
Hence, the surface area of shape so formed is 855cm2.
APPEARS IN
संबंधित प्रश्न
A cubical block of side 7 cm is surmounted by a hemisphere. What is the greatest diameter the hemisphere can have? Find the surface area of the solid. [Use `pi = 22/7`]
Water in a canal, 5·4 m wide and 1·8 m deep, is flowing with a speed of 25 km/hour. How much area can it irrigate in 40 minutes, if 10 cm of standing water is required for irrigation?
From a solid right circular cylinder of height 2.4 cm and radius 0.7 cm, a right circular cone of same height and same radius is cut out. Find the total surface area of the remaining solid.
The diameters of the lower and upper ends of a bucket in the form of a frustum of a cone are 10 cm and 30 cm respectively. If its height is 24 cm, find:
1) The area of the metal sheet used to make the bucket.
2) Why we should avoid the bucket made by ordinary plastic? [Use π = 3.14]
The perimeters of the ends of a frustum of a right circular cone are 44 cm and 33 cm. If the height of the frustum be 16 cm, find its volume, the slant surface and the total surface.
A wooden toy is in the shape of a cone mounted on a cylinder, as shown in the figure. The total height of the toy is 26 cm, while the height of the conical part is 6 cm. The diameter of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm. The conical part and the cylindrical part are respectively painted red and white. Find the area to be painted by each of these colours. `["Take" pi = 22/7]`

A spherical ball of radius 3 cm is melted and recast into three spherical balls. The radii of two of these balls are 1.5 cm and 2 cm. Find the radius of the third ball.
In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here?
Three metallic cubes whose edges are 3 cm, 4 cm and 5 cm, are melted and recast into a single large cube. Find the edge of the new cube formed.
There are two identical solid cubical boxes of side 7 cm. From the top face of the first cube a hemisphere of diameter equal to the side of the cube is scooped out. This hemisphere is inverted and placed on the top of the second cube’s surface to form a dome. Find
- the ratio of the total surface area of the two new solids formed
- volume of each new solid formed.
