Question

A block of mass m moves on a horizontal circle against the wall of a cylindrical room of radius R. The floor of the room on which the block moves is smooth but the friction coefficient between the wall and the block is μ. The block is given an initial speed v₀. As a function of the speed v writes
(a) the normal force by the wall on the block,
(b) the frictional force by a wall, and
(c) the tangential acceleration of the block.
(d) Integrate the tangential acceleration \[\left( \frac{dv}{dt} = v\frac{dv}{ds} \right)\] to obtain the speed of the block after one revolution.

Answer 1

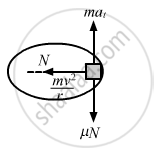
Given:
Radius of the room = R 
Mass of the block = m
(a) Normal reaction by the wall on the block = N = \[\frac{m v^2}{R}\]

(b) Force of frictional by the wall = \[\mu N = \frac{\mu m v^2}{R}\]

(c) Let a_t be the tangential acceleration of the block.
From figure, we get :

\[- \frac{\mu m v^2}{R} = m a_t \]

\[ \Rightarrow a_t = - \frac{\mu v^2}{R}\]

(d) \[\text { On using a }= \frac{dv}{dt} = v\frac{dv}{ds}, \text { we get : } \]

\[v\frac{dv}{ds} = \frac{\mu v^2}{R}\]

\[ \Rightarrow ds = - \frac{R}{\mu}\frac{dv}{v}\]

\[\text { Integrating both side, we get : } \]

\[s = - \frac{R}{\mu}\text{In v + c }\]

\[\text{ At, s = 0, v = v}_0 \]

\[\text{ So, c} = \frac{R}{\mu}\text{ In v}_0 \]

\[ \Rightarrow s = - \frac{R}{\mu}\text{ In}\frac{v}{v_0}\]

\[ \Rightarrow \frac{v}{\text{v}_0} = e^{- \frac{\mu \text{s}}{R}} \]

\[ \Rightarrow \text{v = v}_0 e^{- \frac{\mu s}{R}} \]

\[\text { For one rotation }, \text { we have : }\]

\[s = 2\pi r\]

\[ \therefore \text{v = v}_0 e^{- 2\pi\mu} \]