Advertisements
Advertisements
Question
A chord 10 cm long is drawn in a circle whose radius is `5sqrt(2)` cm. Find the areas of both the segments.
Solution
Let O be the centre of the circle and AB be the chord.
Consider Δ OAB.
OA = OB = `5sqrt(2) "cm"`
OA2 + OB = 50 + 50 = 100
Now,
`sqrt(100) = 10 "cm" = "AB"`
Thus, ΔOAB is a right isosceles triangle.
Thus we have :
Area of Δ OAB `=1/2xx5sqrt(2)xx5sqrt(2) = 25 "cm"^2`
Area of the minor segment = Area of the sector - Area of the triangle
`=(90/360xxpixx(5sqrt(2))^2) - 25`
= 14.25 cm2
Area of the major segment = Area of the circle -- Area of the minor segment
`= pixx(5sqrt(2))^2-14.25`
= 142.75 cm2
APPEARS IN
RELATED QUESTIONS
Find the area of the sector whose arc length and radius are 14 cm and 6 cm respectively.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. [Use `pi = 22/7`]
A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see the given figure). Find
- The area of that part of the field in which the horse can graze.
- The increase in the grazing area of the rope were 10 m long instead of 5 m. [Use π = 3.14]

AB is a chord of circle with centre O and radius 4cm. AB is length of 4cm. Find the area of sector of the circle formed by chord AB
AB is the diameter of a circle, centre O. C is a point on the circumference such that ∠COB = 𝜃. The area of the minor segment cutoff by AC is equal to twice the area of sector BOC.Prove that `"sin"theta/2. "cos"theta/2= pi (1/2−theta/120^@)`
In the given figure, if O is the centre of the circle, PQ is a chord. \[\angle\] POQ = 90°, area of shaded region is 114 cm2 , find the radius of the circle. \[\pi\] = 3.14)

The area of the shaded portion in the following figure is equal to the area of.
In figure, ABCD is a trapezium with AB || DC, AB = 18 cm, DC = 32 cm and distance between AB and DC = 14 cm. If arcs of equal radii 7 cm with centres A, B, C and D have been drawn, then find the area of the shaded region of the figure.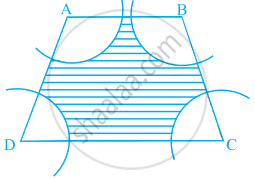
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
The length of the arc of a circle of radius 14 cm which subtends an angle of 60° at the centre of the circle is ______.
