Advertisements
Advertisements
Question
Find the area of the sector whose arc length and radius are 14 cm and 6 cm respectively.
Solution
Length of arc, S = 14 cm
Radius of circle, r = 6 cm
`"S" = rθ ⇒ θ = "S"/r = 14/6`radians
`14/6"radians"=180/pixx14/6=(420/pi)@`
`"Area of the sector "=theta/360xxpir^2=420/(360xxpi)xxpir^2=7/6xx36=42"cm"^2`
APPEARS IN
RELATED QUESTIONS
Find the area of the minor segment of a circle of radius 14 cm, when its central angle is 60˚. Also find the area of the corresponding major segment.[use π=22/7]
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle. [Use π = 3.14 and `sqrt3 = 1.73`]
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
The area of sector of circle of radius 2cm is 𝜋cm2. Find the angle contained by the sector.
The area of sector of circle of radius 5cm is 5𝜋 cm2. Find the angle contained by the sector.
A sector is cut-off from a circle of radius 21 cm the angle of sector is 120°. Find the length of its arc and its area.
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
In the given figure, O is the centre of the circle. m ( arc PQR) = 60° OP = 10 cm. Find the area of the shaded region.( \[\pi\]= 3.14, \[\sqrt{3}\]= 1.73)

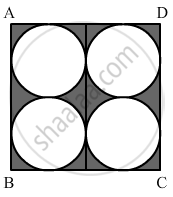
In the given figure, find the area of the shaded region, where ABCD is a square of side 14 cm and all circles are of the same diameter.
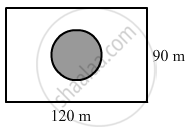
A park is in the form of a rectangle 120 m by 90 m. At the centre of the park there is a circular lawn as shown in the figure. The area of the park excluding the lawn is 2950 m2. Find the radius of the circular lawn.
In following figure , C is a point on the minor arc AB of the circle with centre O . Given ∠ ACB = p° , ∠ AOB = q° , express q in terms of p. Calculate p if OACB is a parallelogram.

In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
If angle of sector is 60°, radius is 3.5 cm then length of the arc is ____________.
If the area of a circle is numerically equal to twice its circumference, then the diameter of the circle is ____________.
The areas of two sectors of two different circles are equal. Is it necessary that their corresponding arc lengths are equal? Why?
Is it true to say that area of a segment of a circle is less than the area of its corresponding sector? Why?
Find the area of the segment of a circle of radius 12 cm whose corresponding sector has a central angle of 60° (Use π = 3.14).
Four circular cardboard pieces of radii 7 cm are placed on a paper in such a way that each piece touches other two pieces. Find the area of the portion enclosed between these pieces.
The length of the arc of a circle of radius 14 cm which subtends an angle of 60° at the centre of the circle is ______.
