Advertisements
Advertisements
प्रश्न
Find the area of the sector whose arc length and radius are 14 cm and 6 cm respectively.
उत्तर
Length of arc, S = 14 cm
Radius of circle, r = 6 cm
`"S" = rθ ⇒ θ = "S"/r = 14/6`radians
`14/6"radians"=180/pixx14/6=(420/pi)@`
`"Area of the sector "=theta/360xxpir^2=420/(360xxpi)xxpir^2=7/6xx36=42"cm"^2`
APPEARS IN
संबंधित प्रश्न
Find the area of sector whose arc length and radius are 10 cm and 5 cm respectively
Find the area of the minor segment of a circle of radius 14 cm, when its central angle is 60˚. Also find the area of the corresponding major segment.[use π=22/7]
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60° [Use `pi = 22/7`]
The area of sector of circle of radius 2cm is 𝜋cm2. Find the angle contained by the sector.
In a circle of radius 35 cm, an arc subtends an angle of 72° at the centre. Find the length of arc and area of sector
A sector is cut-off from a circle of radius 21 cm the angle of sector is 120°. Find the length of its arc and its area.
A sector of 56° cut out from a circle contains area of 4.4 cm2. Find the radius of the circle
In the given figure, if A is the centre of the circle. \[\angle\] PAR = 30°, AP = 7.5, find the area of the segment PQR. (\[\pi\] = 3.14)

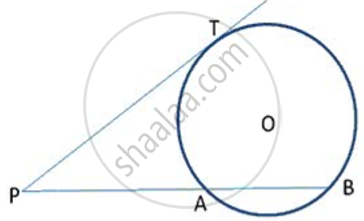
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
Find the area of a sector of a circle having radius 6 cm and length of the arc 15 cm.
The number of revolutions made by a circular wheel of radius 0.7m in rolling a distance of 176m is ______.
If the length of an arc of a circle of radius r is equal to that of an arc of a circle of radius 2r, then the angle of the corresponding sector of the first circle is double the angle of the corresponding sector of the other circle. Is this statement false? Why?
The areas of two sectors of two different circles are equal. Is it necessary that their corresponding arc lengths are equal? Why?
Find the area of a sector of a circle of radius 28 cm and central angle 45°.
Find the area of the minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 60°.
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
Find the difference of the areas of a sector of angle 120° and its corresponding major sector of a circle of radius 21 cm.
The length of the arc of a circle of radius 14 cm which subtends an angle of 60° at the centre of the circle is ______.
Find the area of the sector of a circle of radius 7 cm and of central angle 90°. Also, find the area of corresponding major sector.
Find the area of the unshaded region shown in the given figure.