Advertisements
Advertisements
Question
A map is drawn to scale of 1:20000. Find: The distance covered by 6cm on the map
Solution
Scale = 1:20000
1cm on the map = 20000cm on the land (as the scale is 1:20000) 1km = 100000cm
`"distance(map)"/"distance(land)"` = Scale
`(6)/("distance(land)" xx (100000)) = (1)/((20000)`
Hence 6cm on map
= `(6 xx 20000)/(100000)`
= 1.2km.
APPEARS IN
RELATED QUESTIONS
In figure, ∠CAB = 90º and AD ⊥ BC. If AC = 75 cm, AB = 1 m and BD = 1.25 m, find AD.
In the following figure, if LM || CB and LN || CD, prove that `("AM")/("AB")=("AN")/("AD")`

Prove that, in a right triangle, the square on the hypotenuse is equal to the sum of the squares on the other two sides.
In ΔABC, ∠ABC = ∠DAC, AB = 8 cm, AC = 4 cm and AD = 5 cm.
- Prove that ΔACD is similar to ΔBCA.
- Find BC and CD.
- Find area of ΔACD : area of ΔABC.

Prove that the area of Δ BCE described on one side BC of a square ABCD is one half the area of the similar Δ ACF described on the diagonal AC.
A model of an aeroplane is made to a scale of 1 : 400. Calculate : the length, in cm, of the model; if the length of the aeroplane is 40 m.
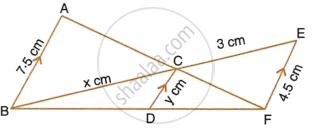
In the figure, given below, AB, CD and EF are parallel lines. Given AB = 7.5 cm, DC = y cm, EF = 4.5 cm, BC = x cm and CE = 3 cm, calculate the values of x and y.
The diagonal AC of a parallelogram ABCD intersects DP at the point Q, where P is any point on side AB. Prove that CQ x PQ = QA x QD.
Find the scale factor in each of the following and state the type of size transformation:
Image length = 8cm, Actual length = 20cm.
ΔABC has been reduced by a scale factor 0.6 to ΔA'B'C'/ Calculate: Length of AB, if A'B' = 5.4cm
