Advertisements
Advertisements
Question
A motorbike, initially at rest, picks up a velocity of 72 kmh−1 over a distance of 40 m. Calculate
- acceleration
- time in which it picks up above velocity.
Solution
Initial velocity = u = 0
Final velocity = v = 72 km/h = `72xx5/18` m/s
v = 20 m/s
Distance = S = 40 m
(i) v2 − u2 = 2aS
(20)2 − (0)2 = 2a (40)
80a = 400
a = `400/80`
a = 5 ms−2
(ii) v = u + at
20 = 0 + 5t
5t = 20
t = `20/5`
t = 4 s
APPEARS IN
RELATED QUESTIONS
Distinguish between acceleration and retardation.
State the type of motion represented by the following sketches in Figures.
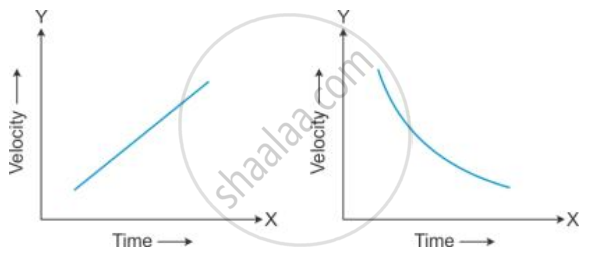
Give an example of each type of motion.
Figure given below shows a velocity-time graph for a car starting from rest. The graph has three parts AB, BC and CD.

(a) Is the magnitude of acceleration higher or lower than that of retardation ? Give a reason .
(b) Compare the magnitude of acceleration and retardation .
A train moving with a velocity of 20 m s-1 is brought to rest by applying brakes in 5 s. Calculate the retardation.
A space craft flying in a straight course with velocity of 75 km s-1 fires its rocket motors for 6.0 s. At the end of this time its speed is 120 km s-1 in the same direction.
Find
(i) The space craft's average acceleration while the motors were firing
(ii) The distance travelled by the space craft in the first 10 s after the rocket motors were started, the motors being in action for only 6 s.
Give an example of an accelerated body, moving with a uniform speed.
From the diagram given below, calculate acceleration.

Diagram is given below shows velocity – time graph of car P and Q, starting from the same place and in the same direction. Calculate the Acceleration of car Q between 2 s – 5 s.

A car starts from rest and it is travelling with a velocity of 20 m/s in 10 s. What is its acceleration?
Assertion: A negative acceleration of a body can be associated with speeding up of the body.
Reason: Increase in the speed of a moving body is independent of its direction of motion.
