Question

A particle of mass m is kept on a fixed, smooth sphere of radius R at a position where the radius through the particle makes an angle of 30° with the vertical. The particle is released from this position. (a) What is the force exerted by the sphere on the particle just after the release? (b) Find the distance travelled by the particle before it loses contact with the sphere. 

Answer 1

(a) When the particle is released from rest, the centrifugal force is zero.

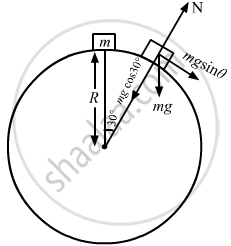
\[\text{N force = mg }\cos \theta = \text{ mg } \cos 30^\circ\]
\[ = \frac{\sqrt{3}}{2} \text{ mg }\]

(b)  Consider that the particle loses contact with the surface at a point whose angle with the horizontal is θ . 

\[\text{So}, \frac{\text{m}\nu^2}{R} = \text{mg}\cos \theta\]
\[ \Rightarrow \nu^2 = \text{Rg} \cos \theta . . . (i)\]
\[\text{Again,} \left( \frac{1}{2} \right) \text{m} \nu^2 = \text{ mg }R \left( \cos 30^\circ- \cos \theta \right)\]
\[ \Rightarrow \nu^2 = 2\text{ Rg }\left( \frac{\sqrt{3}}{2} - \cos \theta \right) . . . (\text{ii})\]

From equations (i) and (ii),

\[\text{ Rg } \cos \theta = 2 \text{ Rg } \left[ \frac{\sqrt{3}}{2} - \cos \theta \right]\]

\[\Rightarrow 3 \cos \theta = \sqrt{3}\]
\[ \Rightarrow \cos \theta = \frac{1}{\sqrt{3}}\]
\[\text{ or }  \theta = \cos^{- 1} \frac{1}{\sqrt{3}}\]

So, the distance travelled by the particle before losing contact,

\[L = R \left( \theta - \frac{\pi}{6} \right)\]

\[ \left[ \text{ because }30^\circ= \left( \frac{\pi}{6} \right) \right]\]

Putting the value of θ, we get:
L = 0.43 R