Advertisements
Advertisements
Question
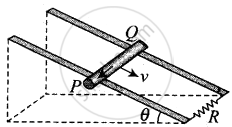
A rod of mass m and resistance R slides smoothly over two parallel perfectly conducting wires kept sloping at an angle θ with respect to the horizontal (Figure). The circuit is closed through a perfect conductor at the top. There is a constant magnetic field B along the vertical direction. If the rod is initially at rest, find the velocity of the rod as a function of time.

Solution
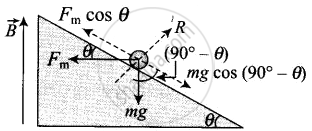
Let us first divide the magnetic field in the components one is along the inclined plane = B sin θ and other component of magnetic field is perpendicular the plane = B cos θ
Now, the conductor moves with speed v perpendicular to B cos θ, component of magnetic field. This causes motional emf across two ends of rod, which is given by = v(B cos θ)d
 (A) |
 (B) |
This makes flow of induced current `i = (v(B cos theta)d)/R` where R is the resistance of rod. Now, current carrying rod experience a magnetic force which is given by `F_m = iBd` (horizontally in backwards direction). Now, the component of magnetic force parallel to the inclined plane in upward direction
`F_(||) = F_m cos θ = -Bd cos θ = ((v(B cos θ)d)/R) Bd cos θ`
Where, `v = (dx)/(dt)`
Also, the component of weight (mg) parallel to the inclined plane along downward direction = mg sin θ.
Now, by Newton's second law of motion
`m (d^2x)/(dl^2) = mg sin θ - (B cos θ d)/R ((dx)/(dt)) xx (Bd) cos θ`
⇒ `(dv)/(dt) = g sin θ - (B^2d^2)/(mR) (cos theta)^2v`
⇒ `(dv)/(dt) + (B^2d^2)/(mR) (cos θ)^2v = g sin θ`
But, this is the linear differential equation.
On solving, we get
`v = ((g sin θ)/(B^2d^2 cos^2 θ))/(mR) + A exp(- (B^2d^2)/(mR) (cos^2 θ)t)`
A is a constant to be determined by initial conditions.
The required expression of velocity as a function of time is given by
= `(mgR sin θ)/(B^2d^2 cos^2θ) (1 - exp (- (B^2d^2)/(mR) (cos^2θ)t))`
APPEARS IN
RELATED QUESTIONS
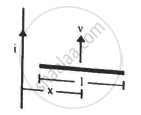
Figure shows a straight, long wire carrying a current i and a rod of length l coplanar with the wire and perpendicular to it. The rod moves with a constant velocity v in a direction parallel to the wire. The distance of the wire from the centre of the rod is x. Find the motional emf induced in the rod.
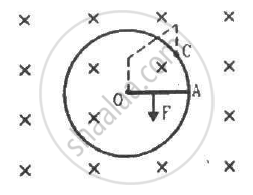
Consider the situation shown in the figure. Suppose the wire connecting O and C has zero resistance but the circular loop has a resistance Runiformly distributed along its length. The rod OA is made to rotate with a uniform angular speed ω as shown in the figure. Find the current in the rod when ∠ AOC = 90°.
A straight conductor of length 2 m moves in a uniform magnetic field of induction 2.5 x `10^-3` T with a velocity. of 4 m/s in a direction perpendicular to its length and also perpendicular to the field. The e.m.f. induced between the ends of the conductor is ______.
A wire of length 50 cm moves with a velocity of 300 m/min, perpendicular to a magnetic field. If the e.m.f. induced in the wire is 2 V, the magnitude of the field in tesla is ______.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.
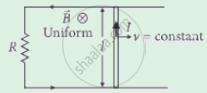
A conducting rod of length l is moving in a transverse magnetic field of strength B with velocity v. The resistance of the rod is R. The current in the rod is ______.
An aeroplane, with its wings spread 10 m, is flying at a speed of 180 km/h in a horizontal direction. The total intensity of earth's field at that part is 2.5 × 10-4 Wb/m2 and the angle of dip is 60°. The emf induced between the tips of the plane wings will be ______.
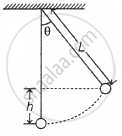
A simple pendulum with a bob of mass m and conducting wire of length L swings under gravity through an angle θ. The component of the earth's magnetic field in the direction perpendicular to the swing is B. Maximum emf induced across the pendulum is ______.
(g = acceleration due to gravity)
Derive an expression for the total emf induced in a conducting rotating rod.
A magnetic flux associated with a coil changes by 0.04 Wb in 0.2 second. The induced emf with coil is ______.
