Advertisements
Advertisements
Question
A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm3 of iron has approximately 8 g mass. [Use π = 3.14]
Solution
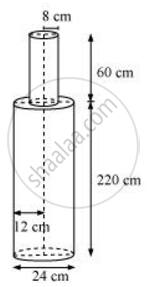
rom the figure, it can be observed that
Height (h1) of larger cylinder = 220 cm
Radius (r1) of larger cylinder = `24/2` = 12 cm
Height (h2) of smaller cylinder = 60 cm
Radius (r2) of smaller cylinder = 8 cm
Total volume of pole = volume of larger cylinder + volume of smaller cylinder
`= pir_1^2h_1+pir_2^2h_2`
`=pi(12)^2xx220+pi(8)^2xx60`
`=pi[144xx220+64xx60]`
= `35520xx3.14`
= 111532.8 cm3
Mass of 1 cm3 iron = 8 g
Mass of 111532.8 cm3 iron
= 111532.8 × 8
= 892262.4 g
= 892.262 kg
APPEARS IN
RELATED QUESTIONS
A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm. Use [π = `22/7`]
A circus tent has cylindrical shape surmounted by a conical roof. The radius of the cylindrical base is 20 m. The heights of the cylindrical and conical portions are 4.2 m and 2.1 m respectively. Find the volume of the tent.
12 spheres of the same size are made from melting a solid cylinder of 16 cm diameter and 2 cm height. The diameter of each sphere is
A solid is in the shape of a cone surmounted on a hemisphere, the radius of each of them being 3.5 cm and the total height of the solid is 9.5 cm. Find the volume of the solid.
A spherical shell of lead, whose external and internal diameters are 24 cm and 18 cm, is melted and recast into a right circular cylinder 37 cm high. Find the diameter of the base of the cylinder.
Three cubes of a metal whose edges are in the ratio 3 : 4 : 5 are melted and converted into a single cube whose diagonal is `12sqrt(3)` cm. Find the edges of the three cubes.
A metallic solid right circular cone is of height 84 cm and the radius of its base is 21 cm. It is melted and recast into a solid sphere. Find the
diameter of the sphere.
A cylindrical vessel with internal diameter 10 cm and height 10.5 cm is full of water. A solid cone of base diameter 7 cm and height 6 cm is completely immersed in water. Find the volume of water
- displaced out of the cylinder
- left in the cylinder.
The diameter of the base of a cone is 42 cm and its volume is 12936 cm3. Its height is
The radii of the base of a cylinder and a cone are in the ratio 3 : 4. If their heights are in the ratio 2 : 3, the ratio between their volumes is
A solid metal cone with base radius 12 cm and height 24 cm is melted to form solid spherical balls of diameter 6 cm each. Find the number of balls formed.
The radius of a solid right circular cylinder increases by 20% and its height decreases by 20%. Find the percentage change in its volume.
The radius of a solid right circular cylinder decreases by 20% and its height increases by 10%. Find the percentage change in its : volume
The given figure shows a solid formed of a solid cube of side 40 cm and a solid cylinder of radius 20 cm and height 50 cm attached to the cube as shown.

Find the volume and the total surface area of the whole solid. [Take π = 3.14].
The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6 cm each and the slant height of the cone is 5 cm. Calculate:
(i) the height of the cone.
(ii) the vol. of the solid.
A solid piece of iron in the form of a cuboid of dimensions 49 cm × 33 cm × 24 cm, is moulded to form a solid sphere. The radius of the sphere is ______.
Three metallic solid cubes whose edges are 3 cm, 4 cm and 5 cm are melted and formed into a single cube. Find the edge of the cube so formed.
A student was asked to make a model shaped like a cylinder with two cones attached to its ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its total length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model.
From a soild cylinder of height 20 cm and diameter 12 cm, a conical cavity of height 8 cm and radius 6 cm is hallowed out. Find the total surface area of the remaining solid.
An ice-cream filled cone having radius 5 cm and height 10 cm is as shown in the figure. Find the volume of the ice-cream in 7 such cones.

