Advertisements
Advertisements
Question
A square water tank has its side equal to 40 m. There are four semi-circular grassy plots all round it. Find the cost of turfing the plot at Rs. 1.25 per square metre (Take π = 3.14).
Solution
It is given that the side of square . `a=40 m`
Since four semicircular grassy plots rounds a square water tank. Then, diameter of semicircular plot is`2r=a`.
So, the radius of semicircle
`r=a/2`
`=40/2`
`20 m`
Area of semicirular plot=`1/2 pir^2`
`= 1/2xx3.14xx20xx20`
`=628 m^2`
Now, the total area of plot is sum of area of four semicircular plots.
`"Total Area of plot"=4xx "Area of semicircle"`
`=4xx628 m^2`
` = 2512 m^2`
Since, The cost of turding the plot per squre meter=`Rs.1.25`
So, The cost turfing 2512 square meter plot= `Rs1.25xx2512`
`= Rs 3140`
APPEARS IN
RELATED QUESTIONS
Find the area of the following circles, given that radius = 5 cm.
Find the cost of polishing a circular table-top of diameter 1.6 m, if the rate of polishing is ₹ 15/m2. (Take π = 3.14)
The base of an isosceles triangle measures 80 cm and its area is 2 `360 . cm^2` Find the perimeter of the triangle.
One side of a rectangle is 12 cm long and its diagonal measure 37 cm. Find the other side and the area of the rectangle.
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
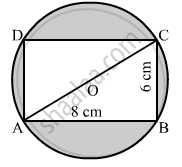
Find the area of the shaded region in the given figure, if ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle.
The area of circle is equal to the sum of the areas of two circles of radii 24 cm and 7 cm. The diameter of the new circle is
Two circles touch externally. The sum of their areas is 130π sq. cm and the distance between their centers is 14 cm. Find the radii of the circles.
The ratio of the area of a circle to the area of its semicircle is
The area of the circle is 154 cm2. The radius of the circle is ______.
