Advertisements
Advertisements
Question
A turn of radius 20 m is banked for the vehicles going at a speed of 36 km/h. If the coefficient of static friction between the road and the tyre is 0.4, what are the possible speeds of a vehicle so that it neither slips down nor skids up?
Solution
Given:
Speed of vehicles = v = 36 km/hr = 10 m/s
Radius = r = 20 m
Coefficient of static friction = μ = 0.4
Let the road be banked with an angle \[\theta\]
We have :
\[\theta = \tan^{- 1} \frac{\text{v}^2}{\text{rg}}\]
\[ = \tan^{- 1} \frac{100}{20 \times 10}\]
\[ = \tan^{- 1} \left( \frac{1}{2} \right)\]
\[ \Rightarrow \text{tan } \theta = 0 . 5\]
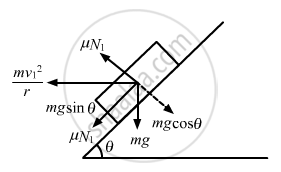
When the car travels at the maximum speed, it slips upward and μN1 acts downward.
Therefore we have :
\[\text{ N}_1 - \text{mg}\cos\theta - \frac{\text{mv}_1^2}{r}\sin\theta = 0 . . . \left( \text{i} \right)\]
\[\mu \text{N}_1 + \text{mg }\sin\theta - \frac{\text{mv}_1^2}{r}\cos\theta = 0 . . . \left(\text{ ii} \right)\]
On solving the above equations, we get :
\[\text{v}_1 = \sqrt{\text{rg}\frac{\mu + \tan\theta}{1 - \mu\tan\theta}}\]
\[ = \sqrt{20 \times 10 \times \frac{0 . 9}{0 . 8}}\]
\[ = 15 \text{ m/s = 54 km/hr }\]
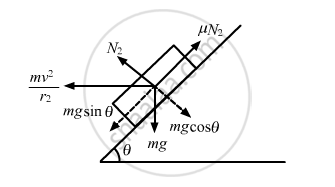
Similarly, for the other case, it can be proved that :
\[\text{v}_2 = \sqrt{\text{rg}\frac{\text{tan}\theta - \mu}{\sqrt{1 - \mu \text{ tan }\theta}}}\]
\[ = \sqrt{20 \times 10 \times \frac{0 . 1}{1 . 2}}\]
\[ = 4 . 08 \text{ m/s = 14 . 7 km/hr}\]
Thus, the possible speeds are between 14.7 km/hr and 54 km/hr so that the car neither slips down nor skids up.
APPEARS IN
RELATED QUESTIONS
A 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
When a particle moves in a circle with a uniform speed
A coin placed on a rotating turntable just slips. If it is placed at a distance of 4 cm from the centre. If the angular velocity of the turntable is doubled, it will just slip at a distance of
Three identical cars A, B and C are moving at the same speed on three bridges. The car A goes on a place bridge, B on a bridge convex upward and C goes on a bridge concave upward. Let FA, FB and FC be the normal forces exerted by the car on the bridges when they are at the middle of bridges.
A train A runs from east to west and another train B of the same mass runs from west to east at the same speed along the equator. A presses the track with a force F1 and B presses the track with a force F2.
A rod of length L is pivoted at one end and is rotated with a uniform angular velocity in a horizontal plane. Let T1 and T2 be the tensions at the points L/4 and 3L/4 away from the pivoted ends.
Assume that the earth goes round the sun in a circular orbit with a constant speed of 30 kms
A car of mass M is moving on a horizontal circular path of radius r. At an instant its speed is v and is increasing at a rate a.
(a) The acceleration of the car is towards the centre of the path.
(b) The magnitude of the frictional force on the car is greater than \[\frac{\text{mv}^2}{\text{r}}\]
(c) The friction coefficient between the ground and the car is not less than a/g.
(d) The friction coefficient between the ground and the car is \[\mu = \tan^{- 1} \frac{\text{v}^2}{\text{rg}.}\]
A hemispherical bowl of radius R is rotated about its axis of symmetry which is kept vertical. A small block is kept in the bowl at a position where the radius makes an angle θ with the vertical. The block rotates with the bowl without any slipping. The friction coefficient between the block and the bowl surface is μ. Find the range of the angular speed for which the block will not slip.
What is the radius of curvature of the parabola traced out by the projectile in the previous problem at a point where the particle velocity makes an angle θ/2 with the horizontal?
A particle of mass 1 kg, tied to a 1.2 m long string is whirled to perform the vertical circular motion, under gravity. The minimum speed of a particle is 5 m/s. Consider the following statements.
P) Maximum speed must be `5sqrt5` m/s.
Q) Difference between maximum and minimum tensions along the string is 60 N.
Select the correct option.
Choose the correct option.
Select correct statement about the formula (expression) of moment of inertia (M.I.) in terms of mass M of the object and some of its distance parameter/s, such as R, L, etc.
A wheel is subjected to uniform angular acceleration about its axis. The wheel is starting from rest and it rotates through an angle θ1, in first two seconds. In the next two seconds, it rotates through an angle θ2. The ratio θ1/θ2 is ____________.
A body is moving along a circular track of radius 100 m with velocity 20 m/s. Its tangential acceleration is 3 m/s2, then its resultant acceleration will be ______.
Angular displacement (θ) of a flywheel varies with time as θ = at + bt2 + ct3 then angular acceleration is given by ____________.
In negotiating curve on a flat road, a cyclist leans inwards by an angle e with the vertical in order to ______.
An engine is moving on a c1rcular path of radius 200 m with speed of 15 m/s. What will be the frequency heard by an observer who is at rest at the centre of the circular path, when engine blows the whistle with frequency 250 Hz?
A person driving a car suddenly applies the brakes on seeing a child on the road ahead. If he is not wearing seat belt, he falls forward and hits his head against the steering wheel. Why?
Which of the following statements is FALSE for a particle moving in a circle with a constant angular speed?
