Advertisements
Advertisements
Question
An aeroplane is flying at a height of 300 m above the ground. Flying at this height, the angles of depression from the aeroplane of two points on both banks of a river in opposite directions are 45° and 60° respectively. Find the width of the river. [Use `sqrt3` = 1⋅732]
Solution
Let CD be the height of the aeroplane above the river at some instant. Suppose A and B be two points on both banks of the river in opposite directions
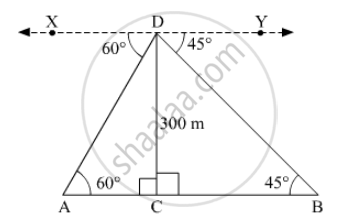
Height of the aeroplane above the river, CD = 300 m
Now,
∠CAD = ∠ADX = 60º (Alternate angles)
∠CBD = ∠BDY = 45º (Alternate angles)
In right ∆ACD,
`tan 60^@ = (CD)/(AC)`
`=> sqrt3 = 300/(AC)`
`=> AC = 300/sqrt3 = 100sqrt3 m`
In right ∆BCD
`tan 45^@ = (CD)/(BC)`
`=> 1 = 300/(BC)`
=> BC = 300 m
∴ Width of the river, AB
= BC + AC
`= 300 + 100sqrt3`
`= 300 + 100 xx 1.73`
= 300 + 173
= 473 m
Thus, the width of the river is 473 m
APPEARS IN
RELATED QUESTIONS
There is a small island in the middle of a 100 m wide river and a tall tree stands on the island. P and Q are points directly opposite to each other on two banks, and in line with the tree. If the angles of elevation of the top of the tree from P and Q are respectively 30º and 45º, find the height of the tree. (Use `sqrt(3)` = 1.732)
The shadow of a tower standing on a level ground is found to be 40 m longer when Sun’s altitude is 30° than when it was 60°. Find the height of the tower.
The angle of elevation of a stationary cloud from a point 2500 m above a lake is 15° and the angle of depression of its reflection in the lake is 45°. What is the height of the cloud above the lake level? (Use tan 15° = 0.268)
An observer 1.5m tall is 30 away from a chimney. The angle of elevation of the top of the chimney from his eye is 60 . Find the height of the chimney.
The angle of elevation of the top of a tower at a distance of 120 m from a point A on the ground is 45 . If the angle of elevation of the top of a flagstaff fixed at the top of the tower, at A is 60 , then find the height of the flagstaff [Use `sqrt(3)` 1.732]
The angle of depression form the top of a tower of a point A on the ground is 30° . On moving a distance of 20 meters from the point A towards the foot of the tower to a point B, the angle of elevation of the top of the tower to from the point B is 60° . Find the height of the tower and its distance from the point A.
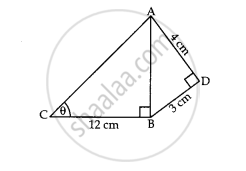
In given figure, AD = 4 cm, BD = 3 cm and CB = 12 cm. The value of tan `theta` is ____________.
An observer `sqrt3` m tall is 3 m away from the pole `2 sqrt3` high. What is the angle of elevation of the top?
The angle of elevation of the top of a vertical tower from a point on the ground is 60°. From another point 10 m vertically above the first, its angle of elevation is 45°. Find the height of the tower.
A person is standing at a distance of 50 m from a temple looking at its top. The angle of elevation is 45°. Find the height of the temple.
