Advertisements
Advertisements
Question
Answer the following question.
Obtain its value for an elastic collision and a perfectly inelastic collision.
Solution
- Consider a head-on collision of two bodies of masses m1 and m2 with respective initial velocities u1 and u2. As the collision is head-on, the colliding masses are along the same line before and after the collision. The relative velocity of approach is given as,
ua = u2 - u1
Let v1 and v2 be their respective velocities after the collision. The relative velocity of recede (or separation) is then vs = v2 – v1
∴ e = `- "v"_"s"/"u"_"a" = - ("v"_2 - "v"_1)/("u"_2 - "u"_1) = ("v"_1 - "v"_2)/("u"_2 - "u"_1)` .....(1) - For a head-on elastic collision, According to the principle of conservation of linear momentum,
Total initial momentum = Total final momentum
∴ m1u1 + m2u2 = m1v1 + m2v2 ...(2)
∴ m1(u1 - v1) = m2(v2 - u2) ......(3)
As the collision is elastic, the total kinetic energy of the system is also conserved.
∴ `1/2 "m"_1"u"_1^2 + 1/2"m"_2"u"_2^2 = 1/2 "m"_1"v"_1^2 + 1/2 "m"_2"v"_2^2` .....(4)
∴ `"m"_1("u"_1^2 - "v"_1^2) = "m"_2("v"_2^2 - "u"_2^2)`
∴ m1(u1 + v1)(u1 - v1) = m2(v2 + u2)(v2 - u2) .....(5)
Dividing equation (5) by equation (3), we get
u1 + v1 = u2 + v2
∴ u2 - u1 = v1 - v2 .....(6)
Substituting this in equation (1),
e = `("v"_1 - "v"_2)/("u"_2 - "u"_1)` = 1 - For a perfectly inelastic collision, the colliding bodies move jointly after the collision, i.e.,
v1 = v2
∴ v1 - v2 = 0
Substituting this in equation (1),
e = 0
APPEARS IN
RELATED QUESTIONS
The rate of change of total momentum of a many-particle system is proportional to the ______ on the system.
State if the following statement is true or false. Give a reason for your answer.
In an elastic collision of two bodies, the momentum and energy of each body is conserved.
Answer carefully, with reason:
In an elastic collision of two billiard balls, is the total kinetic energy conserved during the short time of collision of the balls (i.e. when they are in contact)?
Answer carefully, with reason:
If the potential energy of two billiard balls depends only on the separation distance between their centres, is the collision elastic or inelastic? (Note, we are talking here of potential energy corresponding to the force during collision, not gravitational potential energy.)
The bob A of a pendulum released from 30° to the vertical hits another bob B of the same mass at rest on a table, as shown in the figure. How high does the bob A rise after the collision? Neglect the size of the bobs and assume the collision to be elastic.

A bullet of mass 0.012 kg and horizontal speed 70 m s–1 strikes a block of wood of mass 0.4 kg and instantly comes to rest with respect to the block. The block is suspended from the ceiling by means of thin wires. Calculate the height to which the block rises. Also, estimate the amount of heat produced in the block.
Which of the following potential energy curves in Fig. cannot possibly describe the elastic collision of two billiard balls? Here r is distance between centres of the balls.

Answer the following question.
Discuss the following as special cases of elastic collisions and obtain their exact or approximate final velocities in terms of their initial velocities.
- Colliding bodies are identical.
- A very heavy object collides on a lighter object, initially at rest.
- A very light object collides on a comparatively much massive object, initially at rest.
Answer the following question.
A bullet of mass m1 travelling with a velocity u strikes a stationary wooden block of mass m2 and gets embedded into it. Determine the expression for loss in the kinetic energy of the system. Is this violating the principle of conservation of energy? If not, how can you account for this loss?
Solve the following problem.
A marble of mass 2m travelling at 6 cm/s is directly followed by another marble of mass m with double speed. After a collision, the heavier one travels with the average initial speed of the two. Calculate the coefficient of restitution.
Explain the characteristics of elastic and inelastic collision.
Arrive at an expression for elastic collision in one dimension and discuss various cases.
What is inelastic collision? In which way it is different from an elastic collision. Mention a few examples in day-to-day life for inelastic collision.
A ball of mass 0.1 kg makes an elastic head-on collision with a ball of unknown mass, initially at rest. If the 0 .1 kg ball rebounds at one-third of its original speed, the mass of the other ball is ______.
A bomb of mass 9 kg explodes into two pieces of mass 3 kg and 6 kg. The velocity of mass 3 kg is 16 m/s, The kinetic energy of mass 6 kg is ____________.
In inelastic collision, ____________.
A mass M moving with velocity 'v' along x-axis collides and sticks to another mass 2M which is moving along Y-axis with velocity 3v. After collision, the velocity of the combination is ______.
A body of mas 'm' moving with speed 3 m/s collides with a body of mass '2m' at rest. The coalesced mass will start to move with a speed of ______.
A smooth sphere of mass 'M' moving with velocity 'u' directly collides elastically with another sphere of mass 'm' at rest. After collision, their final velocities are V' and V respectively. The value of V is given by ______.
Two blocks M1 and M2 having equal mass are free to move on a horizontal frictionless surface. M2 is attached to a massless spring as shown in figure. Iniially M2 is at rest and M1 is moving toward M2 with speed v and collides head-on with M2.
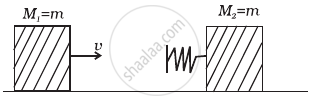
- While spring is fully compressed all the KE of M1 is stored as PE of spring.
- While spring is fully compressed the system momentum is not conserved, though final momentum is equal to initial momentum.
- If spring is massless, the final state of the M1 is state of rest.
- If the surface on which blocks are moving has friction, then collision cannot be elastic.
In an elastic collision of two billiard balls, which of the following quantities remain conserved during the short time of collision of the balls (i.e., when they are in contact).
- Kinetic energy.
- Total linear momentum?
Give reason for your answer in each case.
Two pendulums with identical bobs and lengths are suspended from a common support such that in rest position the two bobs are in contact (Figure). One of the bobs is released after being displaced by 10° so that it collides elastically head-on with the other bob.

- Describe the motion of two bobs.
- Draw a graph showing variation in energy of either pendulum with time, for 0 ≤ t ≤ 2T, where T is the period of each pendulum.
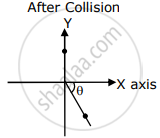
A ball of mass 10 kg moving with a velocity of 10`sqrt3` ms–1 along the X-axis, hits another ball of mass 20 kg which is at rest. After collision, the first ball comes to rest and the second one disintegrates into two equal pieces. One of the pieces starts moving along Y-axis at a speed of 10 m/s. The second piece starts moving at a speed of 20 m/s at an angle θ (degree) with respect to the X-axis.
The configuration of pieces after the collision is shown in the figure.
The value of θ to the nearest integer is ______.
A ball is thrown upwards from the foot of a tower. The ball crosses the top of tower twice after an interval of 4 seconds and the ball reaches ground after 8 seconds, then the height of tower is ______ m. (g = 10 m/s2)
An alpha-particle of mass m suffers 1-dimensional elastic collision with a nucleus at rest of unknown mass. It is scattered directly backwards losing, 64% of its initial kinetic energy. The mass of the nucleus is ______.
Three identical blocks A, B and C are placed on horizontal frictionless surface. The blocks A and C are at rest. But A is approaching towards B with a speed 10 m/s. The coefficient of restitution for all collision is 0.5. The speed of the block C just after the collision is ______.

