Advertisements
Advertisements
Question
Check whether AD is bisector of ∠A of ∆ABC of the following
AB = 5 cm, AC = 10 cm, BD = 1.5 cm and CD = 3.5 cm
Solution
In ∆ABC, AB = 5 cm, AC = 10 cm, BD = 1.5 cm, CD = 3.5 cm
`"BD"/"DC" = 1.5/3.5 = 15/35 = 3/7`
`"AB"/"AC" = 5/10 = 1/2`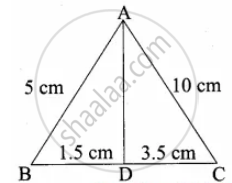
`"BD"/"DC" ≠ "AB"/"AC"`
∴ AD is not a bisector of ∠A.
APPEARS IN
RELATED QUESTIONS
In ∆ABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x – 7, DB = 5x – 3, AE = 4x – 3 and EC = 3x – 1, find the value of x
In ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 12 cm, AD = 8 cm, AE = 12 cm and AC = 18 cm
If PQ || BC and PR || CD prove that `"QB"/"AQ" = "DR"/"AR"`
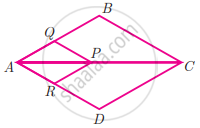
Rhombus PQRB is inscribed in ΔABC such that ∠B is one of its angle. P, Q and R lie on AB, AC and BC respectively. If AB = 12 cm and BC = 6 cm, find the sides PQ, RB of the rhombus.
ABCD is a quadrilateral in which AB = AD, the bisector of ∠BAC and ∠CAD intersect the sides BC and CD at the points E and F respectively. Prove that EF || BD.
Construct a ∆PQR in which the base PQ = 4.5 cm, ∠R = 35° and the median from R to RG is 6 cm.
Construct a ∆ABC such that AB = 5.5 cm, ∠C = 25° and the altitude from C to AB is 4 cm
Draw a triangle ABC of base BC = 5.6 cm, ∠A = 40° and the bisector of ∠A meets BC at D such that CD = 4 cm
ST || QR, PS = 2 cm and SQ = 3 cm. Then the ratio of the area of ∆PQR to the area of ∆PST is

Two circles intersect at A and B. From a point, P on one of the circles lines PAC and PBD are drawn intersecting the second circle at C and D. Prove that CD is parallel to the tangent at P.
