Advertisements
Advertisements
Question
Compute the median for the following data:
| Marks | No. of students |
| More than 150 | 0 |
| More than 140 | 12 |
| More than 130 | 27 |
| More than 120 | 60 |
| More than 110 | 105 |
| More than 100 | 124 |
| More than 90 | 141 |
| More than 80 | 150 |
Solution
| Marks | Class Internal | frequency (f.) |
No. of students |
| More than 80 | 80-90 | 9 | 9 |
| More than 90 | 90-100 | 17 | 26 |
| More than 100 | 100-110 | 19 | 45 |
| More than 110 | 110-120 | 45 | 90 |
| More than 120 | 120-130 | 33 | 123 |
| More than 130 | 130-140 | 15 | 138 |
| More than 140 | 140-150 | 12 | 150 |
| More than 150 | 150-160 | 0 | 150 |
| N = 150 |
We have, N = 150
∴ `"N"/2 = 150/2 = 75`
Thus, the cumulative frequency just greater than 75 is 90 and the corresponding class is 110-120.
Therefore, 110-120 is the median class.
l = 110, f = 45, F = 45 and h = 10
∴ Median = `l + {(N/2-F)/f} × h`
∴ Median = `110 + {(75 - 45)/45} × 10`
∴ Median = `110 + (30)/45 × 10`
∴ Median = `110 + (300)/45`
∴ Median = 110 + 6.67
∴ Median = 116.67 (approx)
Hence, the median is 116.67.
APPEARS IN
RELATED QUESTIONS
Below is the given frequency distribution of words in an essay
| Number of Words | Number of Candidates |
| 600 – 800 | 8 |
| 800 – 1000 | 22 |
| 1000 – 1200 | 40 |
| 1200 – 1400 | 18 |
| 1400 - 1600 | 12 |
Find the mean number of words written.
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 years.
| Age (in years) | Number of policy holders |
| Below 20 | 2 |
| 20 - 25 | 4 |
| 25 - 30 | 18 |
| 30 - 35 | 21 |
| 35 - 40 | 33 |
| 40 - 45 | 11 |
| 45 - 50 | 3 |
| 50 - 55 | 6 |
| 55 - 60 | 2 |
For a certain frequency distribution, the values of Assumed mean (A) = 1300, `sumf_id_i` = 900 and `sumfi` = 100. Find the value of mean (`barx`) .
From the following data, find:
Upper quartile
25, 10, 40, 88, 45, 60, 77, 36, 18, 95, 56, 65, 7, 0, 38 and 83
The weight of 60 boys are given in the following distribution table:
| Weight (kg) | 37 | 38 | 39 | 40 | 41 |
| No. of boys | 10 | 14 | 18 | 12 | 6 |
Find:
- Median
- Lower quartile
- Upper quartile
- Inter-quartile range
Given below is the number of units of electricity consumed in a week in a certain locality:
| Class | 65 – 85 | 85 – 105 | 105 – 125 | 125 – 145 | 145 – 165 | 165 – 185 | 185 – 200 |
| Frequency | 4 | 5 | 13 | 20 | 14 | 7 | 4 |
Calculate the median.
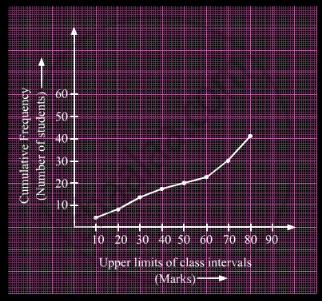
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
If 35 is removed from the data: 30, 34, 35, 36, 37, 38, 39, 40, then the median increased by
The median of the following observations 11, 12, 14, (x – 2), (x + 4), (x + 9), 32, 38, 47 arranged in ascending order is 24. Find the value of x and hence find the mean.
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 year.
| Age (in years) | Number of policy holders |
| Below 20 | 2 |
| Below 25 | 6 |
| Below 30 | 24 |
| Below 35 | 45 |
| Below 40 | 78 |
| Below 45 | 89 |
| Below 50 | 92 |
| Below 55 | 98 |
| Below 60 | 100 |
