Advertisements
Advertisements
Question
Construct a triangle ABC, such that AB= 6 cm, BC= 7.3 cm and CA= 5.2 cm. Locate a point which is equidistant from A, B and C.
Solution
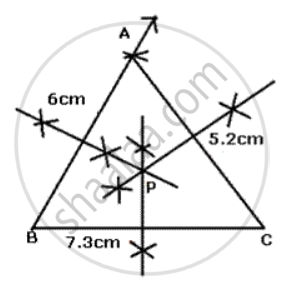
Steps of construction:
(i) Draw a line segment BC= 7.3 cm.
(ii) With Bas centre and radius 6 cm draw an arc.
(iii) With C as centre and radius 5.2 cm draw another arc which intersects the first arc at A.
(iv) Join AB and AC.
(v) Draw perpendicuIar bisector of BC , AB and AC.
In triangIe ABC, P is the point of intersection of AB , AC and BC.
Therefore, PA = PB, PB = PC, PC = PA.
Thus, circum-centre of a triangle is the point which is equidistant from all its vertices.
APPEARS IN
RELATED QUESTIONS
Draw an angle ABC = 75°. Find a point P such that P is at a distance of 2 cm from AB and 1.5 cm from BC.
Construct a rhombus ABCD whose diagonals AC and BD are 8 cm and 6 cm respectively. Find by construction a point P equidistant from AB and AD and also from C and D.
In Δ PQR, s is a point on PR such that ∠ PQS = ∠ RQS . Prove thats is equidistant from PQ and QR.
In given figure 1 ABCD is an arrowhead. AB = AD and BC = CD. Prove th at AC produced bisects BD at right angles at the point M

In Δ ABC, B and Care fixed points. Find the locus of point A which moves such that the area of Δ ABC remains the same.
Describe completely the locus of point in the following cases:
Midpoint of radii of a circle.
Describe completely the locus of points in the following cases:
Centre of a circle of varying radius and touching the two arms of ∠ ABC.
Construct a triangle BPC given BC = 5 cm, BP = 4 cm and .
i) complete the rectangle ABCD such that:
a) P is equidistant from AB and BCV
b) P is equidistant from C and D.
ii) Measure and record the length of AB.
State and draw the locus of a point equidistant from two given parallel lines.
Ruler and compasses only may be used in this question. All construction lines and arcs must be clearly shown, and be of sufficient length and clarity to permit assessment.
(i) Construct a ΔABC, in which BC = 6 cm, AB = 9 cm and ∠ABC = 60°.
(ii) Construct the locus of the vertices of the triangles with BC as base, which are equal in area to ΔABC.
(iii) Mark the point Q, in your construction, which would make ΔQBC equal in area to ΔABC, and isosceles.
(iv) Measure and record the length of CQ.
