Advertisements
Advertisements
Question
Draw an inscribing circle of a regular hexagon of side 5.8 cm.
Solution
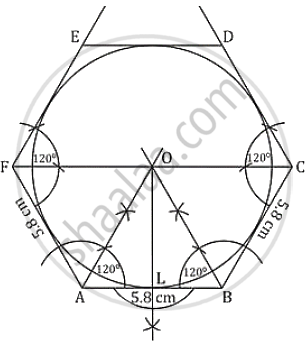
Steps of construction:
- Draw a line segment AB = 5.8 cm.
- At A and B, draw rays making an angle of 120° each and cut off AF = BC = 5.8 cm.
- Again F and C, draw rays making an angle of 120° each and cut off FE = CD = 5.8 cm.
- Join DE. Then ABCDEF is the regular hexagon.
- Draw the bisectors of ∠A and ∠B intersecting each other at O.
- From O, draw OL ⊥ AB.
- With centre O and radius OL, draw a circle which touches the sides of the hexagon.
This is the required in circle of the hexagon.
APPEARS IN
RELATED QUESTIONS
Draw a circle of radius 4 cm. Draw two tangents to the circle inclined at an angle of 60° to each other.
Draw a circle of radius 4.5 cm. Draw two tangents to this circle so that the angle between the tangents is 60°.
Draw a circle of radius 3.5 cm. Mark a point P outside the circle at a distance of 6 cm from the centre. Construct two tangents from P to the given circle. Measure and write down the length of one tangent.
Draw a circle with center O and radius 4 cm. Draw any diameter AB of this circle. Construct tangents to the circle at each of the two end points of the diameter AB.
Write the steps of construction for drawing a pair of tangents to a circle of radius 3 cm , which are inclined to each other at an angle of 60° .
Draw a circle of radius 32 cm. Draw a tangent to the circle making an angle 30° with a line passing through the centre.
A park is of the shape of a circle of diameter 7 m. It is surrounded by a path of width of 0·7 m. Find the expenditure of cementing the path, if its cost is Rs 110 per sq. m ?
Draw an isosceles triangle with sides 6 cm, 4 cm, and 6 cm. Construct the incircle of the triangle. Also, write the steps of construction.
There is a circle with center O. P is a point from where only one tangent can be drawn to this circle. What can we say about P?
Construct a pair of tangents to a circle of radius 3 cm which are inclined to each other at an angle of 60°.
