Advertisements
Advertisements
Question
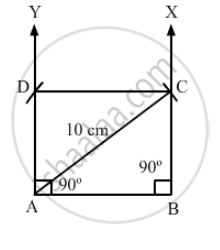
Draw a rectangle whose one side measures 8 cm and the length of each of whose diagonals is 10 cm.
Solution
(i) Draw a side AB, equal to 8 cm.
(ii) With A as the centre, draw an arc of length 10 cm.
(iii) Draw\[\angle\]ABX = 90° which intersects the arc at C.
(iv)Draw\[\angle\]= 90° .
(v) With C as the centre, draw an arc of length 8 cm.
(vi) Join CD.
Thus, ABCD is the required rectangle.
APPEARS IN
RELATED QUESTIONS
Explain why a rectangle is a convex quadrilateral.
Which of the following statement is true for a rectangle?
It has all its sides of equal length.
Which of the following statement is true for a rectangle?
Its diagonals are perpendicular and bisect each other.
Which of the following statement true for a square?
Its diagonals are equal to its sides.
The sides of a rectangle are in the ratio 4 : 5. Find its sides if the perimeter is 90 cm.
ABCD is a rectangle, if ∠BPC = 124°
Calculate:
- ∠BAP
- ∠ADP

All squares are rectangles.
If diagonals of a quadrilateral are equal, it must be a rectangle.
In a rectangle ABCD, AB = 25 cm and BC = 15. In what ratio does the bisector of ∠C divide AB?
PQRS is a rectangle. The perpendicular ST from S on PR divides ∠S in the ratio 2:3. Find ∠TPQ.
