Advertisements
Advertisements
Question
During the medical check-up of 35 students of a class, their weights were recorded as follows:
| Weight (in kg | Number of students |
| Less than 38 | 0 |
| Less than 40 | 3 |
| Less than 42 | 5 |
| Less than 44 | 9 |
| Less than 46 | 14 |
| Less than 48 | 28 |
| Less than 50 | 32 |
| Less than 52 | 35 |
Draw a less than type ogive for the given data. Hence obtain the median weight from the graph verify the result by using the formula.
Solution
The given cumulative frequency distributions of less than type are
|
Weight (in kg upper class limits |
Number of students (cumulative frequency) |
| Less than 38 | 0 |
| Less than 40 | 3 |
| Less than 42 | 5 |
| Less than 44 | 9 |
| Less than 46 | 14 |
| Less than 48 | 28 |
| Less than 50 | 32 |
| Less than 52 | 35 |
Taking upper class limits on x-axis and their respective cumulative frequencies on y-axis, its ogive can be drawn as follows.

Here, n = 35
So n/2 = 17.5
Mark the point A whose ordinate is 17.5 and its x-coordinate is 46.5. Therefore, median of this data is 46.5
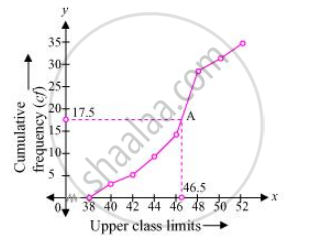
It can be observed that the difference between two consecutive upper class limits is 2. The class marks with their respective frequencies are obtained as below
| Weight (in kg) | Frequency (f) | Cumulative frequency |
| Less than 38 | 0 | 0 |
| 38 − 40 | 3 − 0 = 3 | 3 |
| 40 − 42 | 5 − 3 = 2 | 5 |
| 42 − 44 | 9 − 5 = 4 | 9 |
| 44 − 46 | 14 − 9 = 5 | 14 |
| 46 − 48 | 28 − 14 = 14 | 28 |
| 48 − 50 | 32 − 28 = 4 | 32 |
| 50 − 52 | 35 − 32 = 3 | 35 |
| Total (n) | 35 |
The cumulative frequency just greater than n/2 (35/2 = 17.5) is 28, belonging to class interval 46 − 48.
Median class = 46 − 48
Lower class limit (l) of median class = 46
Frequency (f) of median class = 14
Cumulative frequency (cf) of class preceding median class = 14
Class size (h) = 2
`"Median" = l + ((n/2-cf)/f)xxh`
= `46 + ((17.5-14)/14)xx2`
= 46+ 3.5/7
= 46.5
Therefore, median of this data is 46.5.
Hence, the value of median is verified.
APPEARS IN
RELATED QUESTIONS
The following table gives production yield per hectare of wheat of 100 farms of a village.
| Production yield (in kg/ha) | 50 − 55 | 55 − 60 | 60 − 65 | 65 − 70 | 70 − 75 | 75 − 80 |
| Number of farms | 2 | 8 | 12 | 24 | 38 | 16 |
Change the distribution to a more than type distribution and draw ogive.
From the following frequency, prepare the ‘more than’ ogive.
| Score | Number of candidates |
| 400 – 450 | 20 |
| 450 – 500 | 35 |
| 500 – 550 | 40 |
| 550 – 600 | 32 |
| 600 – 650 | 24 |
| 650 – 700 | 27 |
| 700 – 750 | 18 |
| 750 – 800 | 34 |
| Total | 230 |
Also, find the median.
From the following data, draw the two types of cumulative frequency curves and determine the median:
| Marks | Frequency |
| 140 – 144 | 3 |
| 144 – 148 | 9 |
| 148 – 152 | 24 |
| 152 – 156 | 31 |
| 156 – 160 | 42 |
| 160 – 164 | 64 |
| 164 – 168 | 75 |
| 168 – 172 | 82 |
| 172 – 176 | 86 |
| 176 – 180 | 34 |
What is the cumulative frequency of the modal class of the following distribution?
| Class | 3 – 6 | 6 – 9 | 9 – 12 | 12 – 15 | 15 – 18 | 18 – 21 | 21 – 24 |
|
Frequency |
7 | 13 | 10 | 23 | 54 | 21 | 16 |
The following is the cumulative frequency distribution ( of less than type ) of 1000 persons each of age 20 years and above . Determine the mean age .
| Age below (in years): | 30 | 40 | 50 | 60 | 70 | 80 |
| Number of persons : | 100 | 220 | 350 | 750 | 950 | 1000 |
The mean of a discrete frequency distribution xi / fi, i = 1, 2, ......, n is given by
In the formula `barx=a+h((sumf_iu_i)/(sumf_i))`, for finding the mean of grouped frequency distribution ui = ______.
If the median of the following frequency distribution is 32.5. Find the values of f1 and f2.
The arithmetic mean of the following frequency distribution is 53. Find the value of k.
| Class | 0-20 | 20-40 | 40-60 | 60-80 | 80-100 |
| Frequency | 12 | 15 | 32 | k | 13 |
If the sum of all the frequencies is 24, then the value of z is:
| Variable (x) | 1 | 2 | 3 | 4 | 5 |
| Frequency | z | 5 | 6 | 1 | 2 |
