Advertisements
Advertisements
Question
Find the median from the following data:
| Class | 1 – 5 | 6 – 10 | 11 – 15 | 16 – 20 | 21 – 25 | 26 – 30 | 31 – 35 | 35 – 40 | 40 – 45 |
| Frequency | 7 | 10 | 16 | 32 | 24 | 16 | 11 | 5 | 2 |
Solution
Converting into exclusive form, we get:
| Class | Frequency (f) | Cumulative Frequency (cf) |
| 0.5 – 5.5 | 7 | 7 |
| 5.5 – 10.5 | 10 | 17 |
| 10.5 – 15.5 | 16 | 33 |
| 15.5 – 20.5 | 32 | 65 |
| 20.5 – 25.5 | 24 | 89 |
| 25.5 – 30.5 | 16 | 105 |
| 30.5 – 35.5 | 11 | 116 |
| 35.5 – 40.5 | 5 | 121 |
| 40.5 – 45.5 | 2 | 123 |
| N = Σ𝑓 = 123 |
Now, N = 123
⇒` N/2` = 61.5.
The cumulative frequency just greater than 61.5 is 65 and the corresponding class is 15.5 – 20.5.
Thus, the median class is 15.5 – 20.5.
∴ l = 15.5, h = 5, f = 32, cf = c.f. of preceding class = 33 and `N/2` = 61.5.
∴ Median,` M = l + {h×((N/2−f)/f)}`
`= 15.5 + {5 × ((61.5 − 33)/32)}`
= 15.5 + 4.45
= 19.95
Hence, median = 19.95.
APPEARS IN
RELATED QUESTIONS
100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows:
| Number of letters | Number of surnames |
| 1 - 4 | 6 |
| 4 − 7 | 30 |
| 7 - 10 | 40 |
| 10 - 13 | 6 |
| 13 - 16 | 4 |
| 16 − 19 | 4 |
Determine the median number of letters in the surnames. Find the mean number of letters in the surnames? Also, find the modal size of the surnames.
Calculate the missing frequency from the following distribution, it being given that the median of the distribution is 24.
| Age in years | 0 - 10 | 10 - 20 | 20 - 30 | 30 - 40 | 40 - 50 |
| No. of persons | 5 | 25 | ? | 18 | 7 |
An incomplete distribution is given below:
| Variable: | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 |
| Frequency: | 12 | 30 | - | 65 | - | 25 | 18 |
You are given that the median value is 46 and the total number of items is 230.
(i) Using the median formula fill up missing frequencies.
(ii) Calculate the AM of the completed distribution.
A student got the following marks in 9 questions of a question paper.
3, 5, 7, 3, 8, 0, 1, 4 and 6.
Find the median of these marks.
The ages of 37 students in a class are given in the following table:
| Age (in years) | 11 | 12 | 13 | 14 | 15 | 16 |
| Frequency | 2 | 4 | 6 | 10 | 8 | 7 |
Compute mean from the following data:
| Marks | 0 – 7 | 7 – 14 | 14 – 21 | 21 – 28 | 28 – 35 | 35 – 42 | 42 – 49 |
| Number of Students | 3 | 4 | 7 | 11 | 0 | 16 | 9 |
Find the correct answer from the alternatives given.
| Distance Covered per litre (km) | 12 - 14 | 14 - 16 | 16 - 18 | 18 - 20 |
| No. of cars | 11 | 12 | 20 | 7 |
The median of the distances covered per litre shown in the above data is in the group . . . . . .
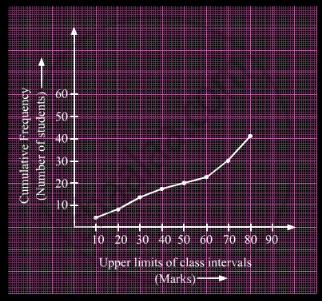
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
Find the median of:
66, 98, 54, 92, 87, 63, 72.
The median of 100 observations grouped in classes of equal width is 25. If the median class interval is 20 –30 and the number of observations less than 20 is 45, then the frequency of median class is ______.
