Advertisements
Advertisements
Question
100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows:
| Number of letters | Number of surnames |
| 1 - 4 | 6 |
| 4 − 7 | 30 |
| 7 - 10 | 40 |
| 10 - 13 | 6 |
| 13 - 16 | 4 |
| 16 − 19 | 4 |
Determine the median number of letters in the surnames. Find the mean number of letters in the surnames? Also, find the modal size of the surnames.
Solution
The cumulative frequencies with their respective class intervals are as follows:
It can be observed that the difference between two consecutive upper class limits is 2. The class marks with their respective frequencies are obtained as below:
| Number of letters | Frequency (f) | Cumulative frequency |
| 1 − 4 | 0 | 6 |
| 4 − 7 | 30 | 30 + 6 = 36 |
| 7 − 10 | 40 | 36 + 40 = 76 |
| 10 − 13 | 16 | 76 + 16 = 92 |
| 13 − 16 | 4 | 92 + 4 = 96 |
| 16 − 19 | 4 | 96 + 4 = 100 |
| Total (n) | 100 |
It can be observed that the cumulative frequency just greater than `n/2 (i.e 100/2 = 50)` is 76, belonging to class interval 7 − 10.
Median class = 7 − 10
Lower limit (l) of median class = 7
Cumulative frequency (cf) of class preceding median class = 36
Frequency (f) of median class = 40
Class size (h) = 3
Median = `l +((n/2-cf)/f) xxh`
= `7+((50-36)/40)xx3`
= `7+(14xx3)/40`
= 8.05
To find the class marks of the given class intervals, the following relation is used.
`"class Mark" = ("Upper class limit + Lower class limit")/2`
Taking 11.5 as assumed mean (a), di, ui, and fiui are calculated according to step deviation method as follows:
|
Number of letters |
Number of surnames fi |
xi |
di = xi− 11.5 |
ui =di/3 |
fiui |
|
1 − 4 |
6 |
2.5 |
− 9 |
− 3 |
−18 |
|
4 − 7 |
30 |
5.5 |
− 6 |
− 2 |
−60 |
|
7 − 10 |
40 |
8.5 |
− 3 |
− 1 |
−40 |
|
10 − 13 |
16 |
11.5 |
0 |
0 |
0 |
|
13 − 16 |
4 |
14.5 |
3 |
1 |
4 |
|
16 − 19 |
4 |
17.5 |
6 |
2 |
8 |
|
Total |
100 |
−106 |
From the table, we obtain
`sumf_i = -106`
`sumf_iu_i = 100`
Mean `barx = a+ ((sumf_iu_i)/(sumf_i))xxh`
= `11.5 + ((-106)/100)xx3`
= 11.5 − 3.18
= 8.32
The data in the given table can be written as:
| Number of letters | Frequency (fi) |
| 1 − 4 | 6 |
| 4 − 7 | 30 |
| 7 − 10 | 40 |
| 10 − 13 | 16 |
| 13 − 16 | 4 |
| 16 − 19 | 4 |
| Total (n) | 100 |
From the table, it can be observed that the maximum class frequency is 40, belonging to class interval 7 − 10.
Modal class = 7 − 10
Lower limit (l) of modal class = 7
Class size (h) = 3
Frequency (f1) of modal class = 40
Frequency (f0) of class preceding the modal class = 30
Frequency (f2) of class succeeding the modal class = 16
Mode = `l+((f_1-f_0)/(2f_1-f_0-f_2))xxh`
= `7+[(40-30)/(2(40)-30-16)]xx3`
= `7 + 10/34 xx 3`
= `7+30/34 = 7.88`
Therefore, median number and mean number of letters in surnames are 8.05 and 8.32, respectively, while modal size of surnames is 7.88.
APPEARS IN
RELATED QUESTIONS
Find the following table gives the distribution of the life time of 400 neon lamps:
| Life time (in hours) | Number of lamps |
| 1500 – 2000 | 14 |
| 2000 – 2500 | 56 |
| 2500 – 3000 | 60 |
| 3000 – 3500 | 86 |
| 3500 – 4000 | 74 |
| 4000 – 4500 | 62 |
| 4500 – 5000 | 48 |
Find the median life time of a lamp.
The mean of following numbers is 68. Find the value of ‘x’. 45, 52, 60, x, 69, 70, 26, 81 and 94. Hence, estimate the median.
The median of the following observations
11, 12, 14, (x - 2), (x + 4), (x + 9), 32, 38, 47 arranged in ascending order is 24.
Find the value of x and hence find the mean.
Following are the lives in hours of 15 pieces of the components of aircraft engine. Find the median:
715, 724, 725, 710, 729, 745, 694, 699, 696, 712, 734, 728, 716, 705, 719.
An incomplete distribution is given below:
| Variable: | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 |
| Frequency: | 12 | 30 | - | 65 | - | 25 | 18 |
You are given that the median value is 46 and the total number of items is 230.
(i) Using the median formula fill up missing frequencies.
(ii) Calculate the AM of the completed distribution.
A student got the following marks in 9 questions of a question paper.
3, 5, 7, 3, 8, 0, 1, 4 and 6.
Find the median of these marks.
The weight of 60 boys are given in the following distribution table:
| Weight (kg) | 37 | 38 | 39 | 40 | 41 |
| No. of boys | 10 | 14 | 18 | 12 | 6 |
Find:
- Median
- Lower quartile
- Upper quartile
- Inter-quartile range
Given below is the number of units of electricity consumed in a week in a certain locality:
| Class | 65 – 85 | 85 – 105 | 105 – 125 | 125 – 145 | 145 – 165 | 165 – 185 | 185 – 200 |
| Frequency | 4 | 5 | 13 | 20 | 14 | 7 | 4 |
Calculate the median.
Calculate the missing frequency from the following distribution, it being given that the median of distribution is 24.
| Class | 0 – 10 | 10 – 20 | 20 – 30 | 30 – 40 | 40 - 50 |
| Frequency | 5 | 25 | ? | 18 | 7 |
In the following data the median of the runs scored by 60 top batsmen of the world in one-day international cricket matches is 5000. Find the missing frequencies x and y.
| Runs scored | 2500 – 3500 | 3500 – 4500 | 4500 – 5500 | 5500 – 6500 | 6500 – 7500 | 7500 - 8500 |
| Number of batsman | 5 | x | y | 12 | 6 | 2 |
If the median of the following frequency distribution is 32.5, find the values of `f_1 and f_2`.
| Class | 0 – 10 | 10 – 20 | 20 – 30 | 30 -40 | 40 – 50 | 50 – 60 | 60 – 70 | Total |
| Frequency | `f_1` |
5 |
9 | 12 | `f_2` | 3 | 2 | 40 |
A data has 25 observations arranged in a descending order. Which observation represents the median?
The following frequency distribution table gives the ages of 200 patients treated in a hospital in a week. Find the mode of ages of the patients.
| Age (years) | Less than 5 | 5 - 9 | 10 - 14 | 15 - 19 | 20 - 24 | 25 - 29 |
| No. of patients | 38 | 32 | 50 | 36 | 24 | 20 |
The median of the following data is 50. Find the values of p and q, if the sum of all the frequencies is 90.
| Marks: | 20 -30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 |
| Frequency: | P | 15 | 25 | 20 | q | 8 | 10 |
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
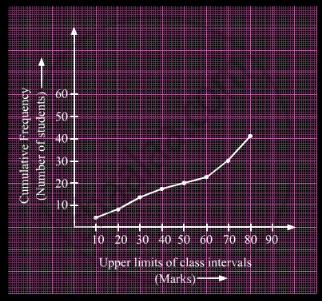
If the median of the data: 6, 7, x − 2, x, 17, 20, written in ascending order, is 16. Then x=
If the difference of Mode and Median of a data is 24, then the difference of median and mean is ______.
In the following table, Σf = 200 and mean = 73. Find the missing frequencies f1, and f2.
| x | 0 | 50 | 100 | 150 | 200 | 250 |
| f | 46 | f1 | f2 | 25 | 10 | 5 |
Find the median of the scores 7, 10, 5, 8, 9.
Find the median of the following frequency distribution:
| x | 10 | 11 | 12 | 13 | 14 | 15 |
| f | 1 | 4 | 7 | 5 | 9 | 3 |
For the following distribution
| Marks | 0 - 10 | 10 - 20 | 20 - 30 | 30 - 40 | 40 - 50 |
| No. of Students | 3 | 9 | 13 | 10 | 5 |
the number of students who got marks less than 30 is?
If 35 is removed from the data, 30, 34, 35, 36, 37, 38, 39, 40 then the median increases by ______.
In a hospital, weights of new born babies were recorded, for one month. Data is as shown:
| Weight of new born baby (in kg) | 1.4 - 1.8 | 1.8 - 2.2 | 2.2 - 2.6 | 2.6 - 3.0 |
| No of babies | 3 | 15 | 6 | 1 |
Then the median weight is?
The abscissa of the point of intersection of the less than type and of the more than type cumulative frequency curves of a grouped data gives its ______.
Consider the data:
| Class | 65 – 85 | 85 – 105 | 105 – 125 | 125 – 145 | 145 – 165 | 165 – 185 | 185 – 205 |
| Frequency | 4 | 5 | 13 | 20 | 14 | 7 | 4 |
The difference of the upper limit of the median class and the lower limit of the modal class is:
The median of an ungrouped data and the median calculated when the same data is grouped are always the same. Do you think that this is a correct statement? Give reason.
The maximum speeds, in km per hour, of 35 cars in a race are given as follows:
| Speed (km/h) | 85 – 100 | 100 – 115 | 115 – 130 | 130 – 145 |
| Number of cars | 5 | 8 | 13 | 9 |
Calculate the median speed.
Find the modal and median classes of the following distribution.
| Class | 0 – 20 | 20 – 40 | 40 – 60 | 60 – 80 | 80 – 100 |
| Frequency | 11 | 22 | 19 | 18 | 7 |
The monthly expenditure on milk in 200 families of a Housing Society is given below:
| Monthly Expenditure (in ₹) |
1000 – 1500 | 1500 – 2000 | 2000 – 2500 | 2500 – 3000 | 3000 – 3500 | 3500 – 4000 | 4000 – 4500 | 4500 – 5000 |
| Number of families | 24 | 40 | 33 | x | 30 | 22 | 16 | 7 |
Find the value of x and also, find the median and mean expenditure on milk.
Consider the following frequency distribution:
| Class | 0 – 6 | 6 – 12 | 12 – 18 | 18 – 24 | 24 – 30 |
| Frequency | 12 | 10 | 15 | 8 | 11 |
The median class is:
A survey conducted on 20 households in a locality by a group of students resulted in the following frequency table for the number of family members in a household:
| Family size | 1 – 3 | 3 – 5 | 5 – 7 | 7 – 9 | 9 – 11 |
| Numbers of Families | 7 | 8 | 2 | 2 | 1 |
Find the median of this data.
The median of first 10 natural numbers is ______.
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 year.
| Age (in years) | Number of policy holders |
| Below 20 | 2 |
| Below 25 | 6 |
| Below 30 | 24 |
| Below 35 | 45 |
| Below 40 | 78 |
| Below 45 | 89 |
| Below 50 | 92 |
| Below 55 | 98 |
| Below 60 | 100 |
