Advertisements
Advertisements
Question
Find the perimeter of the rectangle whose length is 40 cm and a diagonal is 41 cm.
Solution
Length of rectangle = 40 cm, Diagonal = 41 cm
Let the breadth of rectangle = x cm
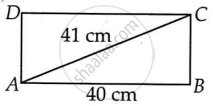
From right angled triangle ΔABC,
AC2 = AB2 + BC2
(41)2 = (40)2 + BC2
BC2 = 1681 – 1600
BC2 = 81
BC = 9
Perimeter of rectangle = 2 (40 + 9)
= 2 × 49
= 98 cm
Hence, perimeter of rectangle = 98 cm
APPEARS IN
RELATED QUESTIONS
ABC is a right-angled triangle, right-angled at A. A circle is inscribed in it. The lengths of the two sides containing the right angle are 5 cm and 12 cm. Find the radius of the circle
In the given figure, AD is a median of a triangle ABC and AM ⊥ BC. Prove that:

`"AC"^2 = "AD"^2 + "BC"."DM" + (("BC")/2)^2`

In ΔMNP, ∠MNP = 90˚, seg NQ ⊥ seg MP, MQ = 9, QP = 4, find NQ.
In the given figure, ∠B = 90°, XY || BC, AB = 12 cm, AY = 8cm and AX : XB = 1 : 2 = AY : YC.
Find the lengths of AC and BC.

ABC is a triangle, right-angled at B. M is a point on BC.
Prove that: AM2 + BC2 = AC2 + BM2
Prove that in a right angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
In the figure below, find the value of 'x'.

A right triangle has hypotenuse p cm and one side q cm. If p - q = 1, find the length of third side of the triangle.
In the given figure, PQ = `"RS"/(3)` = 8cm, 3ST = 4QT = 48cm.
SHow that ∠RTP = 90°.
The foot of a ladder is 6 m away from its wall and its top reaches a window 8 m above the ground. Find the length of the ladder.
