Advertisements
Advertisements
Question
Find the points of discontinuity of the function f, where `f(x) = {{:(sinx",", 0 ≤ x ≤ pi/4),(cos x",", pi/4 < x < pi/2):}`
Solution
Clearly f(x) is defined at all points of `[0, pi/2]`
Case (i) Let x0 ∈ `[0, pi/4]`
`lim_(x -> x_0) f(x) = lim_(x -> x_0) sin x`
= sin x0
`f(x_0)` = sin x0
∴ `lim_(x -> x_0) f(x) = f(x_0)`
Hence f(x) is continuous at x = x0.
Since x0 is an arbitrary point of `[0, pi/4]`
f(x) is continuous at all poin of `[0, pi/4]`
Case (ii) Let x0 ∈ `[pi/4, pi/2]`
`lim_(x -> x_0) f(x) = lim_(x -> x_0) cos x`
= cos x0
`f(x_0)` = cos x0
∴ `lim_(x -> x_0) f(x) = f(x_0)`
Hence, f(x) is continuous at x = x0.
Since x0 is an arbitrary point of `[pi/4, pi/2]`
f(x) is continuous at all points of `[pi/4, pi/2]`
Hence, f (x) is continuous at all points `[0, pi/2]`.
APPEARS IN
RELATED QUESTIONS
Prove that f(x) = 2x2 + 3x - 5 is continuous at all points in R
Examine the continuity of the following:
`sinx/x^2`
Examine the continuity of the following:
`(x^2 - 16)/(x + 4)`
Find the points of discontinuity of the function f, where `f(x) = {{:(4x + 5",", "if", x ≤ 3),(4x - 5",", "if", x > 3):}`
At the given point x0 discover whether the given function is continuous or discontinuous citing the reasons for your answer:
x0 = 3, `f(x) = {{:((x^2 - 9)/(x - 3)",", "if" x ≠ 3),(5",", "if" x = 3):}`
For what value of `alpha` is this function `f(x) = {{:((x^4 - 1)/(x - 1)",", "if" x ≠ 1),(alpha",", "if" x = 1):}` continuous at x = 1?
Let `f(x) = {{:(0",", "if" x < 0),(x^2",", "if" 0 ≤ x ≤ 2),(4",", "if" x ≥ 2):}`. Graph the function. Show that f(x) continuous on `(- oo, oo)`
A function f is defined as follows:
`f(x) = {{:(0, "for" x < 0;),(x, "for" 0 ≤ x ≤ 1;),(- x^2 +4x - 2, "for" 1 ≤ x ≤ 3;),(4 - x, "for" x ≥ 3):}`
Is the function continuous?
Which of the following functions f has a removable discontinuity at x = x0? If the discontinuity is removable, find a function g that agrees with f for x ≠ x0 and is continuous on R.
`f(x) = (x^2 - 2x - 8)/(x + 2), x_0` = – 2
Which of the following functions f has a removable discontinuity at x = x0? If the discontinuity is removable, find a function g that agrees with f for x ≠ x0 and is continuous on R.
`f(x) = (3 - sqrt(x))/(9 - x), x_0` = 9
State how continuity is destroyed at x = x0 for the following graphs.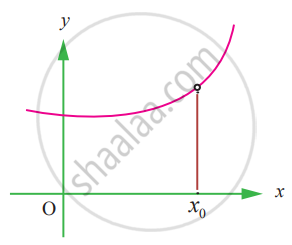
State how continuity is destroyed at x = x0 for the following graphs.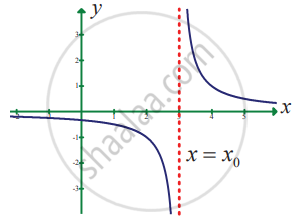
State how continuity is destroyed at x = x0 for the following graphs.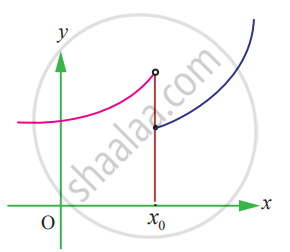
Choose the correct alternative:
Let the function f be defined by `f(x) = {{:(3x, 0 ≤ x ≤ 1),(-3 + 5, 1 < x ≤ 2):}`, then
Choose the correct alternative:
The value of `lim_(x -> "k") x - [x]`, where k is an integer is
Choose the correct alternative:
Let f : R → R be defined by `f(x) = {{:(x, x "is irrational"),(1 - x, x "is rational"):}` then f is
Choose the correct alternative:
The function `f(x) = {{:((x^2 - 1)/(x^3 + 1), x ≠ - 1),("P", x = -1):}` is not defined for x = −1. The value of f(−1) so that the function extended by this value is continuous is
