Advertisements
Advertisements
Question
For a reaction A + B ⟶ P, the rate is given by
Rate = k [A] [B]2
What is the overall order of reaction if A is present in large excess?
Solution 1
If A is present in large excess, then the reaction will be independent of the concentration of A and will be dependent only on the concentration of B. As [B]2 will be the only determining factor in the order of reaction, the overall order of the reaction will be two.
Solution 2
If A is present in large excess, then the rate of the reaction will be independent of A and will depend only on the concentration of B. The overall rate of the reaction will be 2.
APPEARS IN
RELATED QUESTIONS
For a reaction : 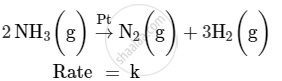
(i) Write the order and molecularity of this reaction.
(ii) Write the unit of k.
For the hydrolysis of methyl acetate in aqueous solution, the following results were obtained :
| t/s | 0 | 30 | 60 |
| [CH3COOCH3] / mol L–1 | 0.60 | 0.30 | 0.15 |
(i) Show that it follows pseudo first order reaction, as the concentration of water remains constant.
(ii) Calculate the average rate of reaction between the time interval 30 to 60 seconds.
(Given log 2 = 0.3010, log 4 = 0.6021)
For the reaction: \[\ce{2A + B → A2B}\] the rate = k[A][B]2 with k = 2.0 × 10−6 mol−2 L2 s−1. Calculate the initial rate of the reaction when [A] = 0.1 mol L−1, [B] = 0.2 mol L−1. Calculate the rate of reaction after [A] is reduced to 0.06 mol L−1.
A reaction is first order in A and second order in B. How is the rate affected on increasing the concentration of B three times?
Write the principle behind the following methods of refining:
Hydraulic washing
Which of the following statements is not correct about order of a reaction.
For a complex reaction:
(i) order of overall reaction is same as molecularity of the slowest step.
(ii) order of overall reaction is less than the molecularity of the slowest step.
(iii) order of overall reaction is greater than molecularity of the slowest step.
(iv) molecularity of the slowest step is never zero or non interger.
Assertion: Rate constants determined from Arrhenius equation are fairly accurate for simple as well as complex molecules.
Reason: Reactant molecules undergo chemical change irrespective of their orientation during collision.
For a first order A → B, the reaction rate at reactant concentration of 0.01 m is found to be 2.0 × 10–5. The half-life period of reaction.
The conversion of molecules A to B follow second order kinetics. If concentration of A is increased to three times, how will it affect the rate of formation of B?
