Advertisements
Advertisements
Question
If θ = 30°, verify that: sin2θ = `(2tanθ)/(1 ++ tan^2θ)`
Solution
Given: θ = 30°
L.H.S.
= sin2θ
= sin2 x 30°
= sin60°
= `sqrt(3)/(2)`
R.H.S.
= `(2tanθ)/(1 + tan^2θ)`
= `(2tan30°)/(1 + tan^2 30°)`
= `(2 xx 1/sqrt(3))/(1 + (1/sqrt(3))^2`
= `(2/sqrt(3))/(1 + 1/3)`
= `((2)/sqrt(3))/(4/3)`
= `(2)/sqrt(3) xx (3)/(4)`
= `sqrt(3)/(2)`
⇒ L.H.S. = R.H.S.
⇒ sin2θ = `(2tanθ)/(1 + tan^2 θ)`.
APPEARS IN
RELATED QUESTIONS
Solve the following equation for A, if sec 2A = 2
Solve for x : sin (x + 10°) = `(1)/(2)`
Solve for x : tan2 (x - 5°) = 3
Find the value of 'A', if cosec 3A = `(2)/sqrt(3)`
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
Find the value 'x', if: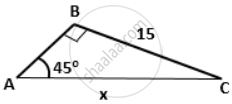
The perimeter of a rhombus is 100 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.
Evaluate the following: `(tan42°)/(cot48°) + (cos33°)/(sin57°)`
Evaluate the following: `(sin0° sin35° sin55° sin75°)/(cos22° cos64° cos58° cos90°)`
Prove the following: `(tan(90° - θ)cotθ)/("cosec"^2 θ)` = cos2θ
