Advertisements
Advertisements
Question
If PQ || BC and PR || CD prove that `"AR"/"AD" = "AQ"/"AB"`

Solution
In ∆ABC, We have PQ || BC
By basic proportionality theorem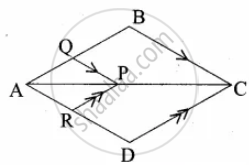
`"AQ"/"AB" = "AP"/"AC"` ...(1)
In ∆ACD, We have PR || CD
Basic proportionality theorem
`"AP"/"AC" = "AR"/"AD"` ...(2)
From (1) and (2) we get
`"AQ"/"AB" = "AR"/"AD"`
or
`"AR"/"AD" = "AQ"/"AB"`
APPEARS IN
RELATED QUESTIONS
In ∆ABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB" = 3/4` and AC = 15 cm find AE
ABCD is a trapezium in which AB || DC and P, Q are points on AD and BC respectively, such that PQ || DC if PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD
In trapezium ABCD, AB || DC, E and F are points on non-parallel sides AD and BC respectively, such that EF || AB. Show that = `"AE"/"ED" = "BF"/"FC"`
DE || BC and CD || EE Prove that AD2 = AB × AF
∠QPR = 90°, PS is its bisector. If ST ⊥ PR, prove that ST × (PQ + PR) = PQ × PR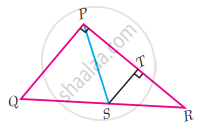
ABCD is a quadrilateral in which AB = AD, the bisector of ∠BAC and ∠CAD intersect the sides BC and CD at the points E and F respectively. Prove that EF || BD.
Construct a ∆PQR in which the base PQ = 4.5 cm, ∠R = 35° and the median from R to RG is 6 cm.
Construct a ∆PQR in which QR = 5 cm, ∠P = 40° and the median PG from P to QR is 4.4 cm. Find the length of the altitude from P to QR.
Draw a triangle ABC of base BC = 5.6 cm, ∠A = 40° and the bisector of ∠A meets BC at D such that CD = 4 cm
An Emu which is 8 feet tall is standing at the foot of a pillar which is 30 feet high. It walks away from the pillar. The shadow of the Emu falls beyond Emu. What is the relation between the length of the shadow and the distance from the Emu to the pillar?
