Advertisements
Advertisements
Question
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.Prove that BO = CO and the ray AO is the bisector of angle BAC.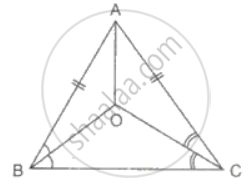
Solution
In ΔABC,
Since AB = AC
∠C = ∠B ...(angles opposite to the equal sides are equal)
BO and CO are angle bisectors of ∠B and ∠C respectively
Hence, ∠ABO = ∠OBC = ∠BCO = ∠ACO
Join AO to meet BC at D
In ΔABO and ΔACO and
AO = AO
AB = AC
∠C = ∠B =
Therefore, ΔBAO ≅ ΔACO ...(SAS criteria)
Hence, ∠BAO = ∠CAO
⇒ AO bisects angle BAC
In ΔABO and ΔACO
and AB = AC
AO = AO
∠BAD = ∠CAD = ...(proved)
ΔBAO ≅ ΔACO ...(SAS criteria)
Therefore,
BO = CO.
APPEARS IN
RELATED QUESTIONS
Complete the congruence statement:
ΔBCA ≅?
ΔQRS ≅?
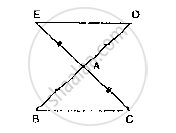
In Fig. 10.22, the sides BA and CA have been produced such that: BA = AD and CA = AE.
Prove that segment DE || BC.
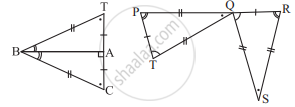
Observe the information shown in pair of triangle given below. State the test by which the two triangles are congruent. Write the remaining congruent parts of the triangles.

From the information shown in the figure,
in ΔPTQ and ΔSTR
seg PT ≅ seg ST
∠PTQ ≅ ∠STR ...[Vertically opposite angles]
∴ ΔPTQ ≅ ΔSTR ...`square` test
∴ `{:("∠TPQ" ≅ square),("and" square ≅ "∠TRS"):}}` ...corresponding angles of congruent triangles
seg PQ ≅ `square` ...corresponding sides of congruent triangles
In the given figure, seg AB ≅ seg CB and seg AD ≅ seg CD. Prove that ΔABD ≅ ΔCBD.

In a triangle, ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB. Prove that: AD = CE.
Prove that:
(i) ∆ ABC ≅ ∆ ADC
(ii) ∠B = ∠D
In ΔABC and ΔPQR and, AB = PQ, BC = QR and CB and RQ are extended to X and Y respectively and ∠ABX = ∠PQY. = Prove that ΔABC ≅ ΔPQR.
In ΔABC, AD is a median. The perpendiculars from B and C meet the line AD produced at X and Y. Prove that BX = CY.
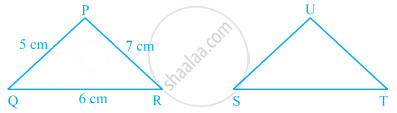
If ∆PQR is congruent to ∆STU (see figure), then what is the length of TU?
Without drawing the triangles write all six pairs of equal measures in the following pairs of congruent triangles.
∆STU ≅ ∆DEF
