Advertisements
Advertisements
Question
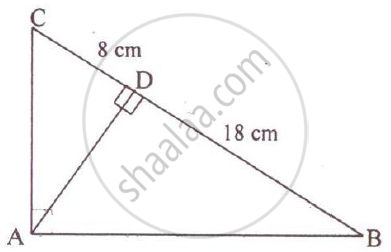
In the adjoining figure, ABC is a right angled triangle with ∠BAC = 90°.
1) Prove ΔADB ~ ΔCDA.
2) If BD = 18 cm CD = 8 cm Find AD.
3) Find the ratio of the area of ΔADB is to an area of ΔCDA.

Solution
1) Let ∠CAD = x
⇒ ∠DAB = 90° - x
∠DAB = 180 - (90° + 90° - x) = x
∠CAD =- ∠DBA .....(1)
In ΔADB and ΔCDA
∠ADB = ∠ADC [each 90°]
∠ABD = ∠CAD [From 1]
∴ ΔADB ~ ΔCDA (AA similarity criterion)
2) Since the corresponding sides of similar triangles are proportional.
`:. (CD)/(AD) = (DA)/(DB)`
`=> AD^2 = DB xx CD`
`=> AD^2 = 18 xx 8`
`=> AD = 12 cm`
3) The ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
So `(Ar(ΔADB))/(Ar(ΔCDA)) = ("AD"^2)/"CD"^2 = 144/64 = 9/4`
Thus, the required ratio is 9: 4.
APPEARS IN
RELATED QUESTIONS
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

Prove that the line segments joining the mid points of the sides of a triangle form four triangles, each of which is similar to the original triangle
E and F are points on the sides PQ and PR, respectively, of a ΔPQR. For the following case, state whether EF || QR.
PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
The diagonals of a quadrilateral ABCD intersect each other at the point O such that `("AO")/("BO") = ("CO")/("DO")`. Show that ABCD is a trapezium.
In the given figure, ∠ABC = 75°, ∠EDC = 75° state which two triangles are similar and by which test? Also write the similarity of these two triangles by a proper one to one correspondence.

In the adjoining figure, the medians BD and CE of a ∆ABC meet at G. Prove that
(i) ∆EGD ∼ ∆CGB and
(ii) BG = 2GD for (i) above.
In ΔABC, MN is drawn parallel to BC. If AB = 3.5cm, AM : AB = 5 : 7 and NC = 2cm, find:
(i) AM
(ii) AC
A model of a ship is made to a scale of 1:500. Find: The length of the ship, if length of the model is 1.2.
In any triangle _______ sides are opposite to equal angles
If ΔABC ∼ ΔDEF and ∠A = 48°, then ∠D = ______.
