Advertisements
Advertisements
Question
In the below fig, if l || m || n and `∠`1 = 60°, find `∠`2.
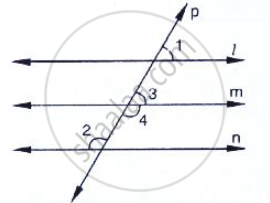
Solution
Since l parallel to m and 𝑝 is the transversal
∴Given: l || m || n, `∠`1 = 60°
To find `∠`2
`∠`1 = `∠`3 = 60° [Corresponding angles]
Now,
`∠`3 and `∠`4 are linear pair of angles
`∠`3 + `∠`4 = 180°
60° + `∠`4 = 180°
`∠`4 = 180° - 60°
`∠`4 = 120°
Also, m || n and P is the transversal
∴`∠`4 = Ð2 =120° [Alternative interior angle]
Hence `∠`2 =120°
APPEARS IN
RELATED QUESTIONS
In the below fig, l, m and n are parallel lines intersected by transversal p at X, Y and Z
respectively. Find ∠1, ∠2 and ∠3.

Prove that the straight lines perpendicular to the same straight line are parallel to one
another.
Which pair of lines in the below fig, is parallel? Given reasons.

In the given figure, if AB || CD, then the value of x is

In the given figure, if l1 || l2, what is the value of y?

In the given figure, if lines l and m are parallel, then x =
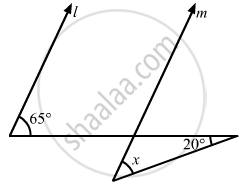
In the given figure, if lines l and m are parallel lines, then x =

In the given figure, if lines l and m are parallel, then the value of x is

In the following figure, if OP || RS, ∠OPQ = 110° and ∠QRS = 130°, then ∠PQR is equal to ______.

A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
