Advertisements
Advertisements
Question
A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
Solution
Given Two lines AB and CD are parallel and intersected by transversal t at P and Q, respectively. Also, EP and FQ are the bisectors of angles ∠APG and ∠CQP, respectively.
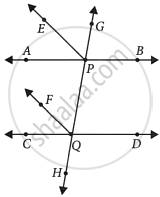
To prove: EP || FQ
Proof: Given, AB || CD
⇒ ∠APG = ∠CQP ...[Corresponding angles]
⇒ `1/2 ∠APG = 1/2 ∠CQP` ...[Dividing both sides by 2]
⇒ ∠EPG = ∠FQP ...[∵ EP and FQ are the bisectors of ∠APG and ∠CQP, respectively]
As these, are the corresponding angles on the transversal line t.
∴ EP || FQ
Hence proved.
APPEARS IN
RELATED QUESTIONS
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then alternate interior angles are equal.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then the interior angles on the same side of the transversal are equal.
If two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 2:3, then the measure of the larger angle is
In the given figure, if l1 || l2, what is the value of y?

In the given figure, if AB || CD, then x =

In the given figure, If line segment AB is parallel to the line segment CD, what is the value of y?

AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (Figure). Show that AP || BQ.
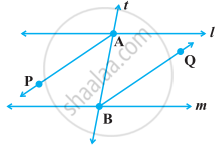
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.

In the following figure, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°