Advertisements
Advertisements
Question
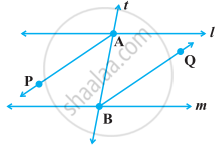
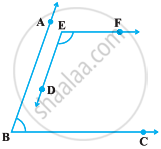
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.
Solution
Given, In the following figure AP || BQ, AP and BQ are the bisectors of alternate interior angles ∠CAB and ∠ABF.
To show l || m
Proof Since, AP || BQ and t is transversal, therefore ∠PAB = ∠ABQ ...[Alternate interior angles]
⇒ 2∠PAB = 2∠ABQ ...[Multiplying both sides by 2]
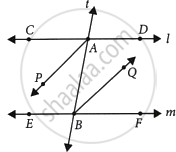
∠CAB = ∠ABF
So, alternate interior angles are equal.
We know that, if two alternate interior angles are equal, then lines are parallel.
Hence, l || m.
APPEARS IN
RELATED QUESTIONS
Which of the following statement are true and false ? Give reason.
If two lines are intersected by a transversal, then corresponding angles are equal.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then the interior angles on the same side of the transversal are equal.
Fill in the blank :
If a transversal intersects a pair of lines in such a way that the sum of interior angles
on the same side of transversal is 180°, then the lines are _______.
In the given figure, if l1 || l2, what is x + y in terms of w and z?
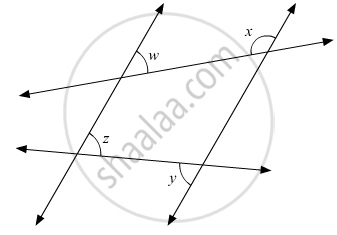
In the given figure, if l1 || l2, what is the value of y?

In the given figure, if AB || CD, then x =

In the given figure, if lines l and m are parallel, then the value of x is

In the following figure, BA || ED and BC || EF. Show that ∠ABC = ∠DEF
[Hint: Produce DE to intersect BC at P (say)].
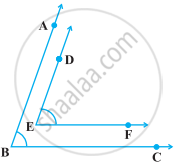
In the following figure, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°
A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
